Advertisements
Advertisements
Question
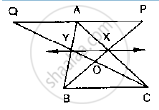
In the below fig. X and Y are the mid-points of AC and AB respectively, QP || BC and
CYQ and BXP are straight lines. Prove that ar (Δ ABP) = ar (ΔACQ).
Solution
Since x and y are the midpoint AC and AB respectively
∴ XY ll BC
Clearly, triangles BYC and BXC are on the same base BC and between the same parallels
XY and BC
∴ area (ΔBYC) = area (BXC)
⇒ area (ΔBYC) = ar (ΔBOC) = ar (ΔBXC) - ar (BOC)
⇒ ar (ΔBOY) = ar (ΔCOX)
⇒ ar ( BOY) + ar (XOY) = ar (ΔCOX) + ar (ΔXOY)
⇒ ar (ΔBXY = ar (ΔCXY)
We observe that the quadrilateral XYAP and XYAQ are on the same base XY and between
the same parallel XY and PQ.
∴ area (quad XYAP ) ar (quad XYPA) ....(2)
Adding (1) and (2), we get
ar (ΔBXY) + ar (quad XYAP) = ar (CXY) + ar (quad XYQA)
⇒ ar (ΔABP) = ar (ΔACQ)
APPEARS IN
RELATED QUESTIONS
P is any point on base BC of ΔABC and D is the mid-point of BC. DE is drawn parallel toPA to meet AC at E. If ar (ΔABC) = 12 cm2, then find area of ΔEPC.
Medians of ΔABC intersect at G. If ar (ΔABC) = 27 cm2, then ar (ΔBGC) =
The perimeter of a triangle ABC is 37 cm and the ratio between the lengths of its altitudes be 6: 5: 4. Find the lengths of its sides.
Let the sides be x cm, y cm, and (37 - x - y) cm. Also, let the lengths of altitudes be 6a cm, 5a cm, and 4a cm.
Find the area of a square, whose side is: 4.5 cm.
Length of a rectangle is 30 m and its breadth is 20 m. Find the increase in its area if its length is increased by 10 m and its breadth is doubled.
Is the area of your belt the same as the area of the postcard? Why or why not?
If each square on this page is equal to 1 square meter of land, how much land will each of her children get? ________ square m
Karunya bought three fields.
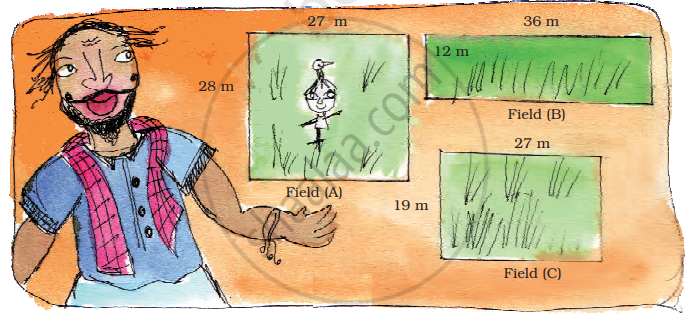
Find the area of all three fields.
- Field (A) ____________ square metre.
- Field (B) ____________ square metre.
- Field (C) ____________ square metre.
Each line gives a story. You have to choose the question which makes the best story problem. The first one is already marked.
- The cost of one book is Rs 47. Sonu buys 23 books.
a) How much money does she have? b) How much money does she pay for the books? c) What is the cost of 47 books?
Find the area of the following figure by counting squares:

