Advertisements
Advertisements
Question
In a convex hexagon, prove that the sum of all interior angle is equal to twice the sum of its exterior angles formed by producing the sides in the same order.
Solution
APPEARS IN
RELATED QUESTIONS
In a quadrilateral, define of the following Adjacent angles .
In a quadrilateral, define of the following Interior .
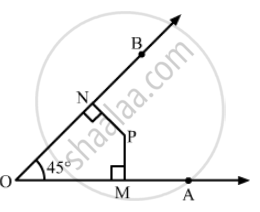
In Fig. 16.20, find the measure of ∠MPN.
The measure of angles of a hexagon are x°, (x − 5)°, (x − 5)°, (2x − 5)°, (2x − 5)°, (2x + 20)°. Find the value of x.
The consecutive sides of a quadrilateral have
In a parallelogram ABCD, its diagonals AC and BD intersect each other at point O.
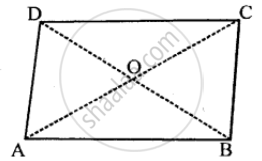
If AC = 12 cm and BD = 9 cm ; find; lengths of OA and OD.
One diagonal of a rectangle is 18 cm. What is the length of its other diagonal?
In a quadrilateral ABCD, ∠A = 72° and ∠C is the supplementary of ∠A. The other two angles are 2x – 10 and x + 4. Find the value of x and the measure of all the angles
ABCD is a rhombus such that ∠ACB = 40º. Then ∠ADB is ______.
In quadrilateral ROPE, the pairs of adjacent angles are ______.
