Advertisements
Advertisements
Question
In the following figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate:
the area of the cross-section.
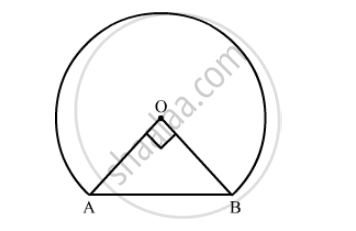
Solution
We have a cross section of a railway tunnel. `ΔOAB`is a right angled isosceles triangle, right angled at O. let OM be perpendicular to AB.
Area of cross section,
`3/4 ("Area of circle")+"Area o"f ΔOAB`
`=(pi)(2)^2(3/4)+1/2(2)(2)`
`=(3pi+2)m^2`
APPEARS IN
RELATED QUESTIONS
Find the length of the hypotenuse of an isosceles right-angled triangle whose area is `200^2` cm . Also, find its perimeter
In the given figure, ΔABC is an equilateral triangle the length of whose side is equal to 10 cm, and ΔADC is right-angled at D and BD= 8cm. Find the area of the shaded region.

In the following figure a square OABC is inscribed in a quadrant OPBQ of a circle. If OA = 21 cm, find the area of the shaded region.

If a square is inscribed in a circle, what is the ratio of the areas of the circle and the square?
How many revolutions a circular wheel of radius r metres makes in covering a distance of s metres?
Area of the largest triangle that can be inscribed in a semi-circle of radius r units is ______.
If the area of the circle is numerically equal to twice its circumference then what is the diameter of the circle?
A rope by which a cow is tethered is increased from 16 m to 23 m. How much additional ground does it have now graze?
The length of the minute hand of a clock is 21 cm. The area swept by the minute hand in 10 minutes is
The area of the circle whose diameter is 21 cm is ____________.
