Advertisements
Advertisements
Question
In the following figure, the square ABCD is divided into five equal parts, all having same area. The central part is circular and the ines AE, GC, BF and HD lie along the diagonals AC and BD of the square. If AB = 22 cm, find:
the circumference of the central part.

Solution
We have a square ABCD.

We have,
`AB=22 cm`
We have to find the perimeter of the triangle. We have a relation as,
Area of circular region=`1/5("Area of" ABCD)`
So,
`pir^2=1/5(22)^2`
`r=22/sqrt5pi`
`=5.56`
So perimeter of the circular region,
`=2pi r`
`=(22)22/7(22/sqrt(5pi))`
APPEARS IN
RELATED QUESTIONS
In the given figure, ABCD is rectangle of dimensions 21 cm × 14 cm. A semicircle is drawn with BC as diameter. Find the area and the perimeter of the shaded region in the figure.

In the following figure, the boundary of the shaded region consists of four semi-circular arcs, the smallest two being equal. If the diameter of the largest is 14 cm and of the smallest is 3.5 cm, find
the length of the boundary.
the area of the shaded region.

In the following figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate:
the perimeter of the cross-section
If the circumference of two circles are in the ratio 2 : 3, what is the ratio of their areas?
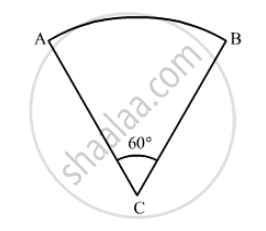
If the adjoining figure is a sector of a circle of radius 10.5 cm, what is the perimeter of the sector? (Take \[\pi = 22/7\])
The radius of a wheel is 0.25 m. The number of revolutions it will make to travel a distance of 11 km will be
If the area of the circle is numerically equal to twice its circumference then what is the diameter of the circle?
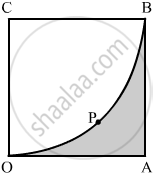
In the given figure, OABC is a square of side 7 cm. If COPB is a quadrant of a circle with centre C find the area of the shaded region.
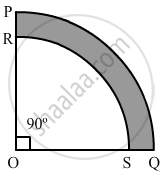
In the given figure, PQRS represents a flower bed. If OP = 21 m and OR = 14 m, find the area of the flower bed.
In the given figure, ABCD is a square each of whose sides measures 28 cm. Find the area of the shaded region.

