Advertisements
Advertisements
Question
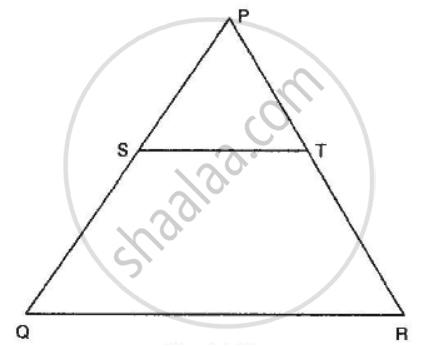
In the given figure, S and T are points on the sides PQ and PR respectively of ∆PQR such that PT = 2 cm, TR = 4 cm and ST is parallel to QR. Find the ratio of the areas of ∆PST and ∆PQR.
Solution
Given: In ΔPQR, S and T are the points on the sides PQ and PR respectively such that PT = 2cm, TR = 4cm and ST is parallel to QR.
To find: Ratio of areas of ΔPST and ΔPQR
\[In ∆ PST and ∆ PQR, \]
\[\angle PST = \angle Q \left( \text{Corresponding angles} \right)\]
\[\angle P = \angle P \left( \text{Common} \right)\]
\[ \therefore ∆ PST ~ ∆ PQR \left( AA \hspace{0.167em} \text{Similarity} \right)\]
Now, we know that the areas of two similar triangles are in the ratio of the squares of the corresponding sides. Therefore,
`(Area(Δ PST))/(Area(Δ PQR))= (PT^2)/(PR^2)`
`(Area(Δ PST))/(Area(Δ PQR))= (PT^2)/(PT+TR)^2`
`(Area(Δ PST))/(Area(Δ PQR))= (2^2)/(2+4)^2`
`(Area(Δ PST))/(Area(Δ PQR))= 4/36=1/9`
APPEARS IN
RELATED QUESTIONS
Diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at the point O. Using similarity criterion for two triangles, show that `"OA"/"OC"="OB"/"OD"`.
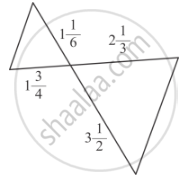
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
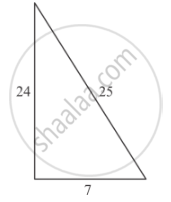
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
Corresponding sides of two triangles are in the ratio 2 : 3. If the area of the smaller triangle is 48 cm2, determine the area of the larger triangle.
There is a staircase as shown in the given figure, connecting points A and B. Measurements of steps are marked in the figure. Find the straight line distance between A and B.

In ∆ABC, ∠C is an obtuse angle. AD ⊥ BC and AB2 = AC2 + 3 BC2. Prove that BC = CD.
In the figure given below DE || BC. If AD = 2.4 cm, DB = 3.6 cm, AC = 5 cm. Find AE.
If the areas of two similar triangles ABC and PQR are in the ratio 9 : 16 and BC = 4.5 cm, what is the length of QR?
If in two triangles ABC and DEF, \[\frac{AB}{DE} = \frac{BC}{FE} = \frac{CA}{FD}\], then
The length of the hypotenuse of an isosceles right triangle whose one side is\[4\sqrt{2} cm\]
