Advertisements
Advertisements
Question
In the following figure, ∠AOB = 90º and ∠ABC = 30º, then ∠CAO is equal to ______.
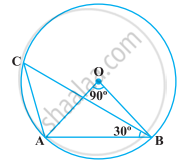
Options
30º
45º
90º
60º
Advertisements
Solution
In the following figure, ∠AOB = 90º and ∠ABC = 30º, then ∠CAO is equal to 60º.
Explanation:
Since, the angle subtended by an arc at the centre is twice the angle subtended by it at any point on the remaining part of the circle.
∴ ∠AOB = 2∠ACB
`\implies` 90° = 2∠ACB ...[∵ ∠AOB = 90°]
`\implies` ∠ACB = 45°
Also, AO = OB ...[Radii of the same circle]
`\implies` ∠ABO = ∠BAO ...(i) [Angles opposite to equal sides are equal]
Now, in ΔOAB, ∠OAB + ∠ABO + ∠BOA = 180° ...[Sum of angles of a triangle is 180°]
∴ ∠OAB + ∠OAB + 90° = 180° ...[From (i)]
`\implies` 2∠OAB = 180° – 90° = 90°
`\implies ∠OAB = 90^circ/2 = 45^circ` ...(ii)
Also, in ΔACB, ∠ACB + ∠CBA + ∠CAB = 180° ...[Sum of angles of a triangle is 180°]
∴ 45° + 30° + ∠CAB = 180° ...[∵ ∠ABC = 30°]
`\implies` ∠CAB = 105°
Since, ∠CAO + ∠OAB = ∠CAB
`\implies` ∠CAO + 45° = 105° ...[From (ii)]
`\implies` ∠CAO = 105° – 45° = 60°
APPEARS IN
RELATED QUESTIONS
PA and PB are tangents from P to the circle with centre O. At point M, a tangent is drawn cutting PA at K and PB at N. Prove that KN = AK + BN.
In fig.. O is the center of the circle and BCD is tangent to it at C. Prove that ∠BAC +
∠ACD = 90°
In the given figure, if arc AB = arc CD, then prove that the quadrilateral ABCD is an isosceles– trapezium (O is the centre of the circle).
If ABCD is a cyclic quadrilateral in which AD || BC (In the given figure). Prove that ∠B = ∠C.
In the given figure, common tangents PQ and RS to two circles intersect at A. Prove that PQ = RS.
The point of concurrence of all angle bisectors of a triangle is called the ______.
Use the figure given below to fill in the blank:
______ is a chord of the circle.
Draw a circle of radius 3.6 cm. In the circle, draw a chord AB = 5 cm. Now shade the minor segment of the circle.
Draw circle with the radii given below.
4 cm
The tangent at a point C of a circle and a diameter AB when extended intersect at P. If ∠PCA = 110°, find ∠CBA see figure
