Advertisements
Advertisements
Question
In the given figure, G is the point of concurrence of medians of ΔDEF. Take point H on ray DG such that D-G-H and DG = GH, then prove that `square`GEHF is a parallelogram.

Solution
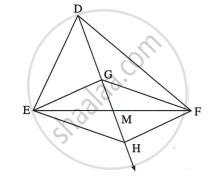
Let, the median side drawn from point D intersects EF at point M.
Point G is the concurrence point.
The concurrence point divides each median in the ratio 2:1.
∴ DG : GM = 2 : 1
∴ `("DG")/("GM") = 2/1`
∴ DG = 2GM ...(i)
∴ DG = GM + MH ...(G-M-H)
∴ 2GM = GM + MH ...[from (i)]
∴ 2GM – GM = MH
∴ GM = MH ...(ii)
In `square`GEHF,
Line GM ≅ Line MH ...[From (ii)]
Line EM ≅ Line MF ...(Point M is the midpoint of line EF)
A quadrilateral is a parallelogram if its diagonals bisect each other.
`square`GEHF is a parallelogram.
APPEARS IN
RELATED QUESTIONS
If the ratio of measures of two adjacent angles of a parallelogram is 1 : 2, find the measures of all angles of the parallelogram.
In the given figure, `square`PQRS and `square`ABCR are two parallelograms. If ∠P = 110° then find the measures of all angles of `square`ABCR.

In the adjacent figure, if seg AB || seg PQ, seg AB ≅ seg PQ, seg AC || seg PR, seg AC ≅ seg PR then prove that, seg BC || seg QR and seg BC ≅ seg QR.
PQRS is a parallelogram whose diagonals intersect at M.
If ∠PMS = 54°, ∠QSR = 25° and ∠SQR = 30° ; find :
(i) ∠RPS
(ii) ∠PRS
(iii) ∠PSR.
In parallelogram ABCD, E is the mid-point of side AB and CE bisects angle BCD. Prove that :
(i) AE = AD,
(ii) DE bisects and ∠ADC and
(iii) Angle DEC is a right angle.
In the following diagram, the bisectors of interior angles of the parallelogram PQRS enclose a quadrilateral ABCD.
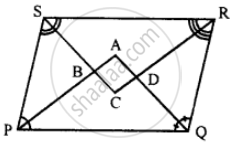
Show that:
(i) ∠PSB + ∠SPB = 90°
(ii) ∠PBS = 90°
(iii) ∠ABC = 90°
(iv) ∠ADC = 90°
(v) ∠A = 90°
(vi) ABCD is a rectangle
Thus, the bisectors of the angles of a parallelogram enclose a rectangle.
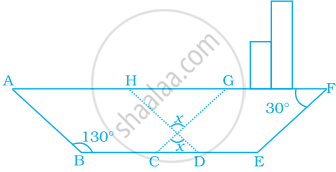
In the following figure of a ship, ABDH and CEFG are two parallelograms. Find the value of x.
Two angles of a quadrilateral are each of measure 75° and the other two angles are equal. What is the measure of these two angles? Name the possible figures so formed.
In parallelogram ABCD, the angle bisector of ∠A bisects BC. Will angle bisector of B also bisect AD? Give reason.
Draw a rough figure of a quadrilateral that is not a parallelogram but has exactly two opposite angles of equal measure.
