Advertisements
Advertisements
Question
O is the circumcentre of the triangle ABC and OD is perpendicular on BC. Prove that ∠BOD = ∠A
Solution
We have to prove that ∠BOD = ∠A
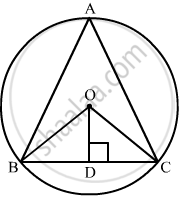
Since, circumcenter is the intersection of perpendicular bisectors of each side of the triangle.
Now according to figure A, B, C are the vertices of ΔABC
In ΔBOC , OD is perpendicular bisector of BC
So, BD = CD
OB = OC (Radius of the same circle)
And,
OD = OD (Common)
Therefore,
We know that angle formed any chord of the circle at the center is twice of the angle formed at the circumference by same chord
Therefore,
` angleBAC = 1/2 angleBOC`
`⇒ angleBAC = 1/2 xx 2 angleBOD`
`⇒ angleBAC = angle BOD`
Therefore,
`angleBOD = angleA`
Hence proved
APPEARS IN
RELATED QUESTIONS
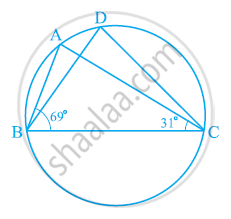
In the given figure, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.
Prove that a diameter of a circle which bisects a chord of the circle also bisects the angle subtended by the chord at the centre of the circle.
If O is the centre of the circle, find the value of x in the following figure:

If O is the centre of the circle, find the value of x in the following figure

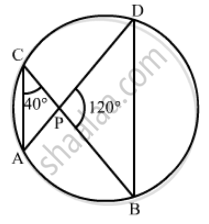
In the given figure, if ∠ACB = 40°, ∠DPB = 120°, find ∠CBD.
In the given figure, two circles intersect at A and B. The centre of the smaller circle is Oand it lies on the circumference of the larger circle. If ∠APB = 70°, find ∠ACB.

In the given figure, two congruent circles with centres O and O' intersect at A and B. If ∠AOB = 50°, then find ∠APB.

In the given figure, AB is a diameter of the circle such that ∠A = 35° and ∠Q = 25°, find ∠PBR.

If ABC is an arc of a circle and ∠ABC = 135°, then the ratio of arc \[\stackrel\frown{ABC}\] to the circumference is ______.
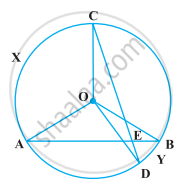
In the following figure, AB and CD are two chords of a circle intersecting each other at point E. Prove that ∠AEC = `1/2` (Angle subtended by arc CXA at centre + angle subtended by arc DYB at the centre).