Advertisements
Advertisements
Question
In the given figure, two circles intersect at A and B. The centre of the smaller circle is Oand it lies on the circumference of the larger circle. If ∠APB = 70°, find ∠ACB.

Solution
Consider the smaller circle whose centre is given as ‘O’.
The angle subtended by an arc at the centre of the circle is double the angle subtended by the arc in the remaining part of the circle.
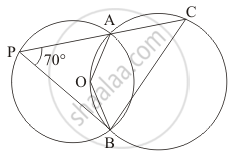
So, here we have
`angle AOB = 2 angle APB `
= 2 (70°)
`angle AOB = 140°`
Now consider the larger circle and the points ‘A’, ‘C’, ‘B’ and ‘O’ along its circumference. ‘ACBO’ form a cyclic quadrilateral.
In a cyclic quadrilateral it is known that the opposite angles are supplementary, meaning that the opposite angles add up to 180°.
`angleAOB + angle ACB` = 180°
`angle ACB = 180° - angle AOB`
`= 180° - 140°`
`angle ACB = 40°`
Hence ,the measure of` ` angle ACB ` is 40° .
APPEARS IN
RELATED QUESTIONS
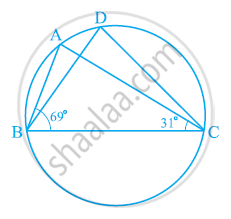
In the given figure, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.
Prove that a diameter of a circle which bisects a chord of the circle also bisects the angle subtended by the chord at the centre of the circle.
If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure
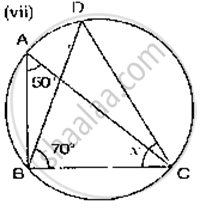
If O is the centre of the circle, find the value of x in the following figures.

In the given figure, it is given that O is the centre of the circle and ∠AOC = 150°. Find ∠ABC.

The chord of a circle is equal to its radius. The angle subtended by this chord at the minor arc of the circle is
In the following figure, ∠ACB = 40º. Find ∠OAB.

