Advertisements
Advertisements
Question
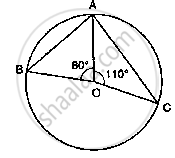
In the following figure, ∠ACB = 40º. Find ∠OAB.

Solution
Given, ∠ACB = 40°
We know that, a segment subtends an angle to the circle is half the angle subtends to the centre.
∴ ∠AOB = 2∠ACB
⇒ `∠ACB = (∠AOB)/2`
⇒ `40^circ = 1/2`∠AOB
⇒ ∠AOB = 80° ...(i) [Both are the radius of a circle]
In ΔAOB, AO = BO
⇒ ∠OBA = ∠OAB ...(ii) [Angles opposite to the equal sides are equal]
We know that, the sum of all three angles in a triangle AOB is 180°.
∴ ∠AOB + ∠OBA + ∠OAB = 180°
⇒ 80° + ∠OAB + ∠OAB = 180° ...[From equations (i) and (ii)]
⇒ 2∠OAB = 180° – 80°
⇒ 2∠OAB = 100°
∴ ∠OAB = `100^circ/2` = 50°
APPEARS IN
RELATED QUESTIONS
In the below fig. O is the centre of the circle. Find ∠BAC.
If O is the centre of the circle, find the value of x in the following figure

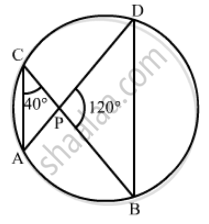
In the given figure, if ∠ACB = 40°, ∠DPB = 120°, find ∠CBD.
In the given figure, it is given that O is the centre of the circle and ∠AOC = 150°. Find ∠ABC.

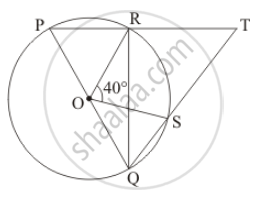
In the given figure, O is the centre of a circle and PQ is a diameter. If ∠ROS = 40°, find ∠RTS.
Prove that the angle in a segment greater than a semi-circle is less than a right angle.
Prove that the line segment joining the mid-point of the hypotenuse of a right triangle to its opposite vertex is half the hypotenuse.
In the given figure, AB is a diameter of the circle such that ∠A = 35° and ∠Q = 25°, find ∠PBR.

In the given figure, if O is the circumcentre of ∠ABC, then find the value of ∠OBC + ∠BAC.

A circle has radius `sqrt(2)` cm. It is divided into two segments by a chord of length 2 cm. Prove that the angle subtended by the chord at a point in major segment is 45°.
