Advertisements
Advertisements
Question
A circle has radius
Solution
Draw a circle having centre O. Let AB = 2 cm be a chord of a circle. A chord AB is divided by the line OM in two equal segments.
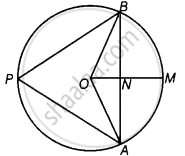
To prove: ∠APB = 45°
Here, AN = NB = 1 cm
And OB =
In ΔONB, OB2 = ON2 + NB2 ...[Use Pythagoras theorem]
⇒
⇒ ON2 = 2 – 1 = 1
⇒ ON = 1 cm ...[Taking positive square root, because distance is always positive]
Also, ∠ONB = 90° ...[ON is the perpendicular bisector of the chord AB]
∴ ∠NOB = ∠NBO = 45°
Similarly, ∠AON = 45°
Now, ∠AOB = ∠AON + ∠NOB
= 45° + 45°
= 90°
We know that, chord subtends an angle to the circle is half the angle subtended by it to the centre.
∴
=
= 45°
Hence proved.
APPEARS IN
RELATED QUESTIONS
Given an arc of a circle, complete the circle.
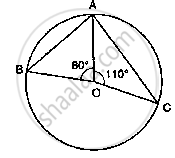
In the below fig. O is the centre of the circle. Find ∠BAC.
If O is the centre of the circle, find the value of x in the following figure:

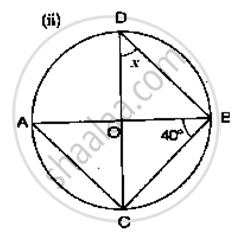
If O is the centre of the circle, find the value of x in the following figure:
If O is the centre of the circle, find the value of x in the following figure

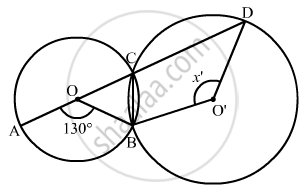
In the given figure, O and O' are centres of two circles intersecting at B and C. ACD is a straight line, find x.
In the given figure, it is given that O is the centre of the circle and ∠AOC = 150°. Find ∠ABC.

In the given figure, P and Q are centres of two circles intersecting at B and C. ACD is a straight line. Then, ∠BQD =

In a circle, the major arc is 3 times the minor arc. The corresponding central angles and the degree measures of two arcs are
The chord of a circle is equal to its radius. The angle subtended by this chord at the minor arc of the circle is
