Advertisements
Advertisements
Question
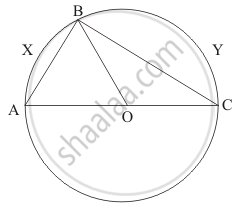
If O is the centre of the circle, find the value of x in the following figure:
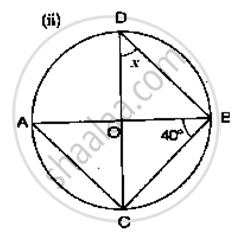
Solution
We have
∠ABC = 40°
∠ACB = 90°
In ∠ABC, by angle sum property
∠CAB + ∠ACB + ∠ABC = 180°
⇒ ∠CAB + 90° + 40° = 180°
⇒ ∠CAB = 180° - 90°
⇒ ∠CAB = 50°
Now,
⇒COB = ∠CAB
⇒x = 50°
APPEARS IN
RELATED QUESTIONS
Prove that the line joining the mid-point of a chord to the centre of the circle passes through the mid-point of the corresponding minor arc.
Given an arc of a circle, complete the circle.
If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure
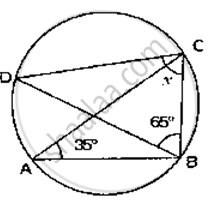
If O is the centre of the circle, find the value of x in the following figures.

O is the circumcentre of the triangle ABC and OD is perpendicular on BC. Prove that ∠BOD = ∠A
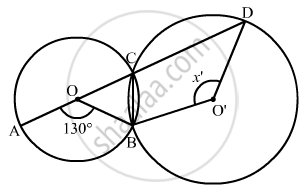
In the given figure, O and O' are centres of two circles intersecting at B and C. ACD is a straight line, find x.
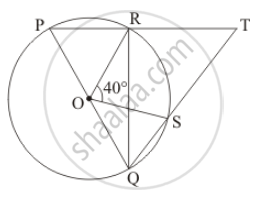
In the given figure, O is the centre of a circle and PQ is a diameter. If ∠ROS = 40°, find ∠RTS.
If the given figure, AOC is a diameter of the circle and arc AXB = \[\frac{1}{2}\] arc BYC. Find ∠BOC.
If arcs AXB and CYD of a circle are congruent, find the ratio of AB and CD.
