Advertisements
Advertisements
Question
Prove that the line joining the mid-point of a chord to the centre of the circle passes through the mid-point of the corresponding minor arc.
Solution
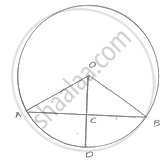
Given: C is the midpoint of chord AB
To prove: D is the midpoint of arc AB Proof:∠
In Δ OAC and ΔOBC
OA=OB [Radius of circle]
OC=OC [Common]
AC=BC [C is the midpoint of AB]
Then, ΔOAC = ΔOBC [By SSS condition]
`∴∠AOC=∠BOC` [ c. p.c.t ]
`⇒m(AD)=M(BD)`
`⇒AD≅ BD `
Here ,D is the midpoint of arc AB
APPEARS IN
RELATED QUESTIONS
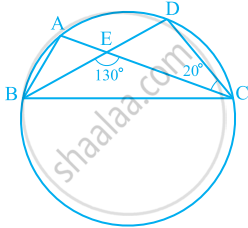
In the given figure, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC = 130° and ∠ECD = 20°. Find ∠BAC.
Given an arc of a circle, show how to complete the circle.
Given an arc of a circle, complete the circle.
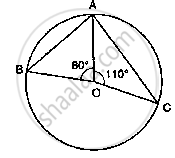
In the below fig. O is the centre of the circle. Find ∠BAC.
If O is the centre of the circle, find the value of x in the following figure:
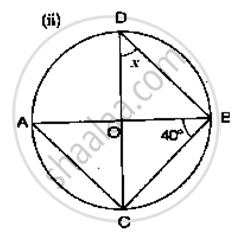
If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figures.

In the given figure, two congruent circles with centres O and O' intersect at A and B. If ∠AOB = 50°, then find ∠APB.

In the given figure, AB is a diameter of the circle such that ∠A = 35° and ∠Q = 25°, find ∠PBR.

