Advertisements
Advertisements
Question
In the given figure, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC = 130° and ∠ECD = 20°. Find ∠BAC.
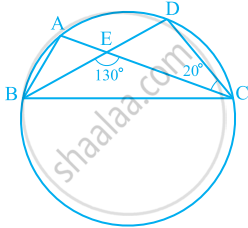
Solution
In ΔCDE,
∠CDE + ∠DCE = ∠CEB ...(Exterior angle)
⇒ ∠CDE + 20° = 130°
⇒ ∠CDE = 110°
However, ∠BAC = ∠CDE ...(Angles in the same segment of a circle)
⇒ ∠BAC = 110°
APPEARS IN
RELATED QUESTIONS
Prove that the line joining the mid-point of a chord to the centre of the circle passes through the mid-point of the corresponding minor arc.
If O is the centre of the circle, find the value of x in the following figure:

If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure
O is the circumcentre of the triangle ABC and OD is perpendicular on BC. Prove that ∠BOD = ∠A
In the given figure, two congruent circles with centres O and O' intersect at A and B. If ∠AOB = 50°, then find ∠APB.

In the given figure, P and Q are centres of two circles intersecting at B and C. ACD is a straight line. Then, ∠BQD =

If ABC is an arc of a circle and ∠ABC = 135°, then the ratio of arc \[\stackrel\frown{ABC}\] to the circumference is ______.
In the following figure, ∠ACB = 40º. Find ∠OAB.

