Advertisements
Advertisements
Question
In the given figure, P and Q are centres of two circles intersecting at B and C. ACD is a straight line. Then, ∠BQD =

Solution
Consider the circle with the centre ‘P’.
The angle subtended by an arc at the centre of the circle is double the angle subtended by the arc in the remaining part of the circle.

So, here we have
`angleACB = (angleAPB )/2`
`=(150°)/2`
`angleACB` = 75°
Since ‘ACD’ is a straight line, we have
`angleACB + angleBCD` = 180°
`angleBCD = 180° - angleACB`
= 180° - 75°
`angleBCD ` = 105°
Now let us consider the circle with centre ‘Q’. Here let ‘E’ be any point on the circumference along the major arc ‘BD’. Now ‘CBED’ forms a cyclic quadrilateral.
In a cyclic quadrilateral it is known that the opposite angles are supplementary, meaning that the opposite angles add up to 180°.
So here,
`angleBCD + angleBED` = 180°
`angleBED = 180° - angleBCD`
= 180° - 105°
`angleBED` = 75°
The angle subtended by an arc at the centre of the circle is double the angle subtended by the arc in the remaining part of the circle.
So, now we have
`angleBQD = 2 angleBED`
=2(75°)
`angleBQD` = 150°
Hence, the measure of `angleBQD` is 150° .
APPEARS IN
RELATED QUESTIONS
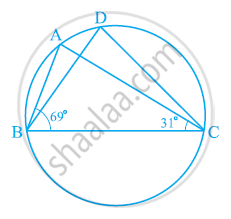
In the given figure, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.
Fill in the blank:
Segment of a circle is the region between an arc and .................. of the circle.
Prove that a diameter of a circle which bisects a chord of the circle also bisects the angle subtended by the chord at the centre of the circle.
O is the circumcentre of the triangle ABC and OD is perpendicular on BC. Prove that ∠BOD = ∠A
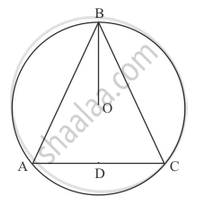
In the given figure, O is the centre of the circle, BO is the bisector of ∠ABC. Show that AB = BC.
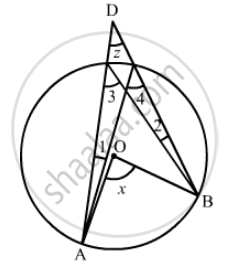
In the given figure, O is the centre of the circle, prove that ∠x = ∠y + ∠z.
In the given figure, two congruent circles with centres O and O' intersect at A and B. If ∠AOB = 50°, then find ∠APB.

In the given figure, A is the centre of the circle. ABCD is a parallelogram and CDE is a straight line. Find ∠BCD : ∠ABE.

In the given figure, if O is the circumcentre of ∠ABC, then find the value of ∠OBC + ∠BAC.

The chord of a circle is equal to its radius. The angle subtended by this chord at the minor arc of the circle is
