Advertisements
Advertisements
Question
In the given figure, A is the centre of the circle. ABCD is a parallelogram and CDE is a straight line. Find ∠BCD : ∠ABE.

Solution
It is given that ‘ABCD’ is a parallelogram. But since ‘A’ is the centre of the circle, the lengths of ‘AB’ and ‘AD’ will both be equal to the radius of the circle.
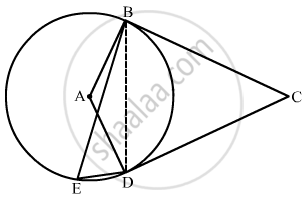
So, we have AB = AD .
Whenever a parallelogram has two adjacent sides equal then it is a rhombus.
So ‘ABCD’ is a rhombus.
Let `angleBDE = x° `.
We know that in a circle the angle subtended by an arc at the centre of the circle is double the angle subtended by the arc in the remaining part of the circle.
By this property we have
`angleBAD = 2 (angleBDE )`
`angleBAD = 2 x°`
In a rhombus the opposite angles are always equal to each other.
So, `angleBAD = angleBCE = 2x°`
Since the sum of all the internal angles in any triangle sums up to 180° in triangle ΔBEC , we have
`angleEBC + angleABE = angleEBC` = 180°
`angleEBC = 180° - angleBEC - angle BCE`
= 180° - x° -2x°
`angleEBS` = 180° - 3x°
In the rhombus ‘ABCD’ since one pair of opposite angles are ‘2x° ’ the other pair of opposite angles have to be (180° - 2x°)
From the figure we see that,
`angleEBC + angle ABE = angleABC `
`angleABE = angleABC - angleEBC `
= 180° - 2x° - (180° - 3x°)
`angleABE` = x°
So now we can write the required ratio as,
`(angleBCD)/(angleABE) = (2x°)/(x°)`
`(angleBCD)/(angleABE) = 2/1`
Hence the ratio between the given two angles is 2: 1 .
APPEARS IN
RELATED QUESTIONS
Fill in the blank:
A circle divides the plane, on which it lies, in ............ parts.
Prove that the line joining the mid-point of a chord to the centre of the circle passes through the mid-point of the corresponding minor arc.
If O is the centre of the circle, find the value of x in the following figure:

If O is the centre of the circle, find the value of x in the following figure

If O is the centre of the circle, find the value of x in the following figure

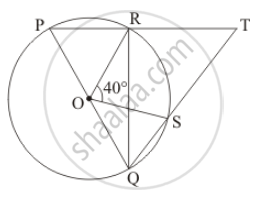
In the given figure, O is the centre of a circle and PQ is a diameter. If ∠ROS = 40°, find ∠RTS.
Prove that the angle in a segment greater than a semi-circle is less than a right angle.
The chord of a circle is equal to its radius. The angle subtended by this chord at the minor arc of the circle is
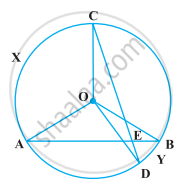
If arcs AXB and CYD of a circle are congruent, find the ratio of AB and CD.
In the following figure, AB and CD are two chords of a circle intersecting each other at point E. Prove that ∠AEC = `1/2` (Angle subtended by arc CXA at centre + angle subtended by arc DYB at the centre).