Advertisements
Advertisements
Question
Given an arc of a circle, show how to complete the circle.
Solution
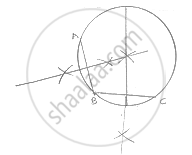
Steps of construction:
(i) Take three point A, B and C on the given Arc
(ii) Join AB and BC
(iii) Draw the perpendicular bisectors of chords AB and BC which interest each other at
point O, then O will required center of the required circle
(iv) Join OA
(v) With center O and radius OA, complete the circle
APPEARS IN
RELATED QUESTIONS
Fill in the blank:
A circle divides the plane, on which it lies, in ............ parts.
Prove that a diameter of a circle which bisects a chord of the circle also bisects the angle subtended by the chord at the centre of the circle.
If O is the centre of the circle, find the value of x in the following figure:
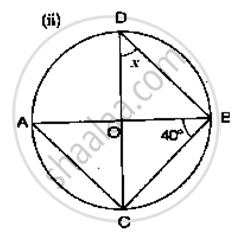
If O is the centre of the circle, find the value of x in the following figure
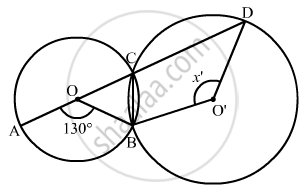
In the given figure, O and O' are centres of two circles intersecting at B and C. ACD is a straight line, find x.
In the given figure, it is given that O is the centre of the circle and ∠AOC = 150°. Find ∠ABC.

In the given figure, two congruent circles with centres O and O' intersect at A and B. If ∠AOB = 50°, then find ∠APB.

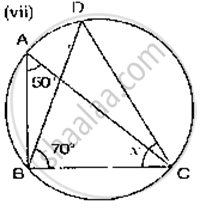
In the following figure, ∠ACB = 40º. Find ∠OAB.

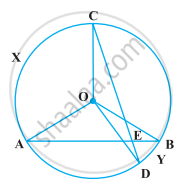
In the following figure, AB and CD are two chords of a circle intersecting each other at point E. Prove that ∠AEC = `1/2` (Angle subtended by arc CXA at centre + angle subtended by arc DYB at the centre).
A circle has radius `sqrt(2)` cm. It is divided into two segments by a chord of length 2 cm. Prove that the angle subtended by the chord at a point in major segment is 45°.
