Advertisements
Advertisements
Question
One of the base angles of an isosceles triangle is 52°. Find its angle of the vertex.
Solution
Each of the base angles of an isosceles
Δ ABC = 52°
∴ ∠B = ∠C = 52°
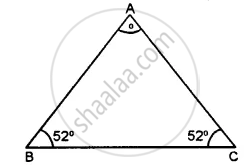
But ∠A + ∠B + ∠C = 180°..........(Angles of a triangle)
⇒ ∠A + 52° + 52° = 180°
⇒ ∠A + 104° = 180°
⇒ ∠A = 180°− 104° = 76°
Hence ∠A = 76°
APPEARS IN
RELATED QUESTIONS
ABC is a triangle in which ∠A — 72°, the internal bisectors of angles B and C meet in O.
Find the magnitude of ∠BOC.
Can a triangle have All angles more than 60°? Justify your answer in case.
Can a triangle have All angles less than 60° Justify your answer in case.
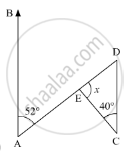
Compute the value of x in the following figure:
Is the following statement true and false :
An exterior angle of a triangle is greater than the opposite interior angles.
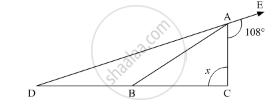
In the given figure, AB divides ∠DAC in the ratio 1 : 3 and AB = DB. Determine the value of x.
State, if the triangle is possible with the following angles :
20°, 70°, and 90°
State, if the triangle is possible with the following angles :
40°, 130°, and 20°
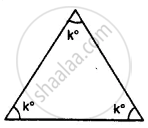
Find the unknown marked angles in the given figure:
The angles of the triangle are 3x – 40, x + 20 and 2x – 10 then the value of x is
