Advertisements
Advertisements
Question
PQRS is a quadrilateral and T and U are points on PS and RS respectively such that PQ = RQ, ∠PQT = ∠RQU and ∠TQS = ∠UQS. Prove that QT = QU.
Solution
∠PQT = ∠RQU .....(i)
∠TQS = ∠UQS .....(ii)
Adding (i) and (ii)
∠PQS = ∠RQS
In ΔPQS and ΔRQS
∠PQS = ∠RQS
PQ = RQ ...(given)
QS = QS ...(common)
Therefore, ΔPQS ≅ ΔRQS ...(SAS criteria)
Hence, ∠QPS = ∠QRS
Now in ΔPQT and ΔRQU
∠QPS = ∠QRS
PQ = RQ ...(given)
∠PQT = ∠RQU ...(given)
Therefore, ΔPQT ≅ ΔRQU ...ASA criteria)
Hence, QT =QU.
APPEARS IN
RELATED QUESTIONS
Prove that the sum of three altitudes of a triangle is less than the sum of its sides.
In the given figure, the measure of ∠B'A'C' is

In the given figure, if AC is bisector of ∠BAD such that AB = 3 cm and AC = 5 cm, then CD =

In the following example, a pair of triangles is shown. Equal parts of triangle in each pair are marked with the same sign. Observe the figure and state the test by which the triangles in each pair are congruent.
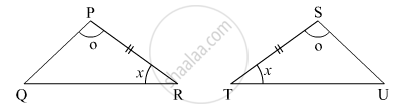
By ______ test
ΔPRQ ≅ ΔSTU
In the pair of triangles given below, the parts shown by identical marks are congruent. State the test and the one-to-one correspondence of vertices by which the triangles in the pair are congruent, the remaining congruent parts.

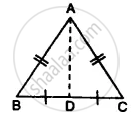
The following figure has shown a triangle ABC in which AB = AC. M is a point on AB and N is a point on AC such that BM = CN.
Prove that: (i) AM = AN (ii) ΔAMC ≅ ΔANB

State, whether the pairs of triangles given in the following figures are congruent or not:


Prove that:
- ∆ ABD ≅ ∆ ACD
- ∠B = ∠C
- ∠ADB = ∠ADC
- ∠ADB = 90°
State, whether the pairs of triangles given in the following figures are congruent or not:

In ΔABC and ΔPQR and, AB = PQ, BC = QR and CB and RQ are extended to X and Y respectively and ∠ABX = ∠PQY. = Prove that ΔABC ≅ ΔPQR.