Advertisements
Advertisements
Question
Solve the following question using appropriate Euclid’s axiom:
Look at the figure. Show that length AH > sum of lengths of AB + BC + CD.

Solution
Given in the question, AB, BC and CD are parts of line.
Then, AB + BC + CD = AD ...(i)
And AD is the part of line AH.
Now, By Euclid’s axiom 5, the whole is greater than the part.
So, AH > AD
That is length AH > sum of length of AB + BC + CD ...[By using (i)]
APPEARS IN
RELATED QUESTIONS
The following statement is true or false? Give reason for your answer.
Only one line can pass through a single point.
In how many points two distinct lines can intersect?
A pyramid is a solid figure, the base of which is ______.
It is known that if x + y = 10 then x + y + z = 10 + z. The Euclid’s axiom that illustrates this statement is ______.
Thales belongs to the country ______.
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have X and Y are the mid-points of AC and BC and AX = CY. Show that AC = BC.
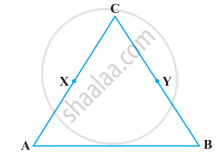
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have BX = `1/2` AB, BY = `1/2` BC and AB = BC. Show that BX = BY.
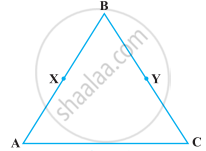
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have ∠1 = ∠2, ∠2 = ∠3. Show that ∠1 = ∠3.
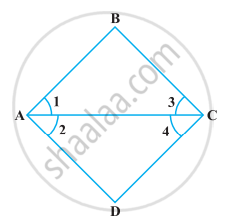
Read the following statements which are taken as axioms:
- If a transversal intersects two parallel lines, then corresponding angles are not necessarily equal.
- If a transversal intersect two parallel lines, then alternate interior angles are equal.
Is this system of axioms consistent? Justify your answer.
The following statement is true or false? Give reason for your answer.
A terminated line can be produced indefinitely on both the sides.
