Advertisements
Advertisements
Question
Solve the following system of inequalities:
`x/(2x + 1) ≥ 1/4, (6x)/(4x - 1) < 1/2`
Solution
From the first inequality, We have `x/(2x + 1) - 1/4 ≥ 0`
⇒ `(2x - 1)/(2x + 1) ≥ 0`
⇒ (2x – 1 ≥ 0 and 2x + 1 > 0) or (2x – 1 ≤ 0 and 2x + 1 < 0) ......[Since 2x + 1 ≠ 0)
⇒ `(x ≥ 1/2 "and" x > - 1/2)` or `(x < 1/2 "and" x < - 1/2)`
⇒ `x ≥ 1/2` or `x < -1/2`
⇒ x ∈ `(-oo, - 1/2) ∪ [1/2, oo)` ....(1)
From the second inequality, We have `(6x)/(4x - 1) - 1/2 < 0`
⇒ `(8x + 1)/(4x - 1) < 0`
⇒ (8x + 1 < 0 and 4x – 1 > 0) or (8x + 1 > 0 and 4x – 1 < 0)
⇒ `(x < - 1/8 "and" x > 1/4)` or `(x > 1/8 "and" x < 1/4)`
⇒ x ∈ `(-1/8, 1/4)` (Since the first is not possible) ...(2)
Note that the common solution of (1) and (2) is null set. Hence, the given system of inequalities has no solution.
APPEARS IN
RELATED QUESTIONS
Solve: 12x < 50, when x ∈ R
Solve: 4x − 2 < 8, when x ∈ R
\[\frac{2\left( x - 1 \right)}{5} \leq \frac{3\left( 2 + x \right)}{7}\]
\[\frac{4 + 2x}{3} \geq \frac{x}{2} - 3\]
\[\frac{1}{x - 1} \leq 2\]
\[\frac{x - 1}{x + 3} > 2\]
Solve
\[\left| \frac{2x - 1}{x - 1} \right| > 2\]
Solve \[\left| x - 1 \right| + \left| x - 2 \right| + \left| x - 3 \right| \geq 6\]
Solve \[\frac{\left| x - 2 \right| - 1}{\left| x - 2 \right| - 2} \leq 0\]
Mark the correct alternative in each of the following:
The inequality representing the following graph is
Mark the correct alternative in each of the following:
The linear inequality representing the solution set given in 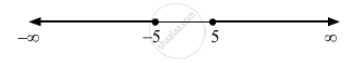
Solve the inequality, 3x – 5 < x + 7, when x is a whole number.
The cost and revenue functions of a product are given by C(x) = 20x + 4000 and R(x) = 60x + 2000, respectively, where x is the number of items produced and sold. How many items must be sold to realise some profit?
Solve for x, |x + 1| + |x| > 3.
If |3x – 7| > 2, then x ______ `5/3` or x ______ 3.
If |x − 1| > 5, then ______.
If |x + 2| ≤ 9, then ______.
State which of the following statement is True or False.
If xy < 0, then x < 0 and y < 0
If x < –5 and x > 2, then x ∈ (– 5, 2)
If `2/(x + 2) > 0`, then x ______ –2.
If p > 0 and q < 0, then p – q ______ p.
If |x + 2| > 5, then x ______ – 7 or x ______ 3.
If – 2x + 1 ≥ 9, then x ______ – 4.
