Advertisements
Online Mock Tests
Chapters
2: Relations and Functions
3: Trigonometric Functions
4: Principle of Mathematical Induction
5: Complex Numbers and Quadratic Equations
▶ 6: Linear Inequalities
7: Permutations and Combinations
8: Binomial Theorem
9: Sequences and Series
10: Straight Lines
11: Conic Sections
12: Introduction to Three Dimensional Geometry
13: Limits and Derivatives
14: Mathematical Reasoning
15: Statistics
16: Probability
![NCERT Exemplar solutions for Mathematics [English] Class 11 chapter 6 - Linear Inequalities NCERT Exemplar solutions for Mathematics [English] Class 11 chapter 6 - Linear Inequalities - Shaalaa.com](/images/mathematics-english-class-11_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
Advertisements
Solutions for Chapter 6: Linear Inequalities
Below listed, you can find solutions for Chapter 6 of CBSE NCERT Exemplar for Mathematics [English] Class 11.
NCERT Exemplar solutions for Mathematics [English] Class 11 6 Linear Inequalities Solved Examples [Pages 100 - 106]
Short Answer
Solve the inequality, 3x – 5 < x + 7, when x is a natural number.
Solve the inequality, 3x – 5 < x + 7, when x is a whole number.
Solve the inequality, 3x – 5 < x + 7, when x is an integer.
Solve the inequality, 3x – 5 < x + 7, when x is a real number.
Solve
Solve |3 – 4x| ≥ 9.
Solve 1 ≤ |x – 2| ≤ 3.
The cost and revenue functions of a product are given by C(x) = 20x + 4000 and R(x) = 60x + 2000, respectively, where x is the number of items produced and sold. How many items must be sold to realise some profit?
Solve for x, |x + 1| + |x| > 3.
Long Answer Type
Solve for x,
Solve the following system of inequalities:
Find the linear inequalities for which the shaded region in the given figure is the solution set.
Objective Type Choose the correct answer from the given four options against each of the Examples 10 to 13 (M.C.Q.)
If
x ∈ [2,
x ∈ (2,
x ∈ (–
x ∈ (–
The length of a rectangle is three times the breadth. If the minimum perimeter of the rectangle is 160 cm, then ______.
Breadth > 20 cm
Length < 20 cm
Breadth x ≥ 20 cm
Length ≤ 20 cm
Solutions of the inequalities comprising a system in variable x are represented on number lines as given below, then ______.![]()
x ∈ (–
x ∈ [–3, 1]
x ∈ (–
x ∈ [–4, 3]
If |x + 3| ≥ 10, then ______.
x ∈ (–13, 7]
x ∈ (–13, 7]
x ∈ (–∞, –13] ∪ [7, ∞)
x ∈ [–∞, –13] ∪ [7, ∞)
State whether the following statement is True or False.
If x > y and b < 0, then bx < by
True
False
State whether the following statement is True or False.
If xy > 0, then x > 0, and y < 0
True
False
State whether the following statement is True or False.
If xy < 0, then x > 0, and y > 0
True
False
State whether the following statement is True or False.
If x > 5 and x > 2, then x ∈ (5, ∞)
True
False
State whether the following statement is True or False.
If |x| < 5, then x ∈ (–5, 5)
True
False
State whether the following statement is True or False.
Graph of x > –2 is
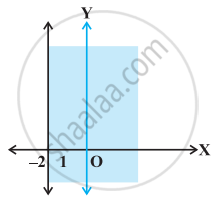
True
False
State whether the following statement is True or False.
Solution set of x – y ≤ 0 is

True
False
Fill in the blanks in the following:
If x ≥ –3, then x + 5 ______ 2.
If –x ≤ –4, then 2x ______ 8.
If
If a < b and c < 0, then
If |x − 1| ≤ 2, then –1 ______ x ______ 3
If |3x – 7| > 2, then x ______
If p > 0 and q < 0, then p + q ______ p.
NCERT Exemplar solutions for Mathematics [English] Class 11 6 Linear Inequalities Exercise [Pages 107 - 113]
Short Answer Type
Solve for x, the inequality given below.
Solve for x, the inequality given below.
Solve for x, the inequality given below.
Solve for x, the inequality given below.
|x − 1| ≤ 5, |x| ≥ 2
Solve for x, the inequality given below.
Solve for x, the inequality given below.
4x + 3 ≥ 2x + 17, 3x – 5 < –2
A company manufactures cassettes. Its cost and revenue functions are C(x) = 26,000 + 30x and R(x) = 43x, respectively, where x is the number of cassettes produced and sold in a week. How many cassettes must be sold by the company to realise some profit?
The water acidity in a pool is considerd normal when the average pH reading of three daily measurements is between 8.2 and 8.5. If the first two pH readings are 8.48 and 8.35, find the range of pH value for the third reading that will result in the acidity level being normal.
A solution of 9% acid is to be diluted by adding 3% acid solution to it. The resulting mixture is to be more than 5% but less than 7% acid. If there is 460 litres of the 9% solution, how many litres of 3% solution will have to be added?
A solution is to be kept between 40°C and 45°C. What is the range of temperature in degree fahrenheit, if the conversion formula is F =
The longest side of a triangle is twice the shortest side and the third side is 2cm longer than the shortest side. If the perimeter of the triangle is more than 166 cm then find the minimum length of the shortest side.
In drilling world’s deepest hole it was found that the temperature T in degree celcius, x km below the earth’s surface was given by T = 30 + 25(x – 3), 3 ≤ x ≤ 15. At what depth will the temperature be between 155°C and 205°C?
Long Answer
Solve the following system of inequalities
Find the linear inequalities for which the shaded region in the given figure is the solution set.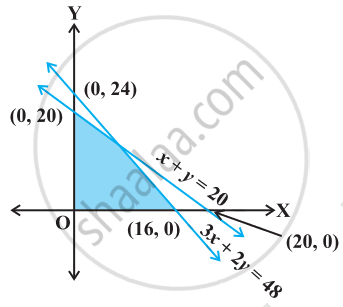
Find the linear inequalities for which the shaded region in the given figure is the solution set.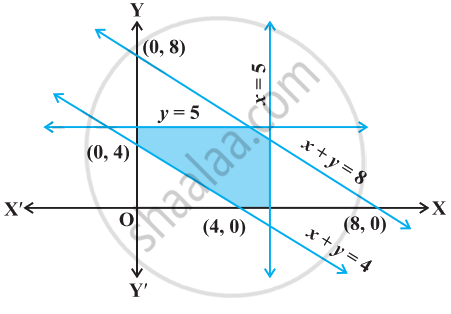
Show that the following system of linear inequalities has no solution x + 2y ≤ 3, 3x + 4y ≥ 12, x ≥ 0, y ≥ 1
Solve the following system of linear inequalities:
3x + 2y ≥ 24, 3x + y ≤ 15, x ≥ 4
Show that the solution set of the following system of linear inequalities is an unbounded region 2x + y ≥ 8, x + 2y ≥ 10, x ≥ 0, y ≥ 0.
Objective Type Questions from 19 to 26
If x < 5, then ______.
–x < –5
–x ≤ –5
–x > –5
–x ≥ –5
Given that x, y and b are real numbers and x < y, b < 0, then ______.
If –3x + 17 < –13, then ______.
x ∈ (10, ∞)
x ∈ [10, ∞)
x ∈ (–∞, 10]
x ∈ [–10, 10)
If x is a real number and |x| < 3, then ______.
x ≥ 3
– 3 < x < 3
x ≤ – 3
– 3 ≤ x ≤ 3
x and b are real numbers. If b > 0 and |x| > b, then ______.
x ∈ (–b, ∞)
x ∈ [–∞, b)
x ∈ (–b, b)
x ∈ (–∞, –b) ∪ (b, ∞)
If |x − 1| > 5, then ______.
x ∈ (–4, 6)
x ∈ [–4, 6]
x ∈ [–∞, –4) ∪ (6, ∞)
x ∈ [–∞, –4) ∪ [6, ∞)
If |x + 2| ≤ 9, then ______.
x ∈ (–7, 11)
x ∈ [–11, 7]
x ∈ (–∞, –7) ∪ (11, ∞)
x ∈ (–∞, –7) ∪ [11, ∞)
The inequality representing the following graph is ______.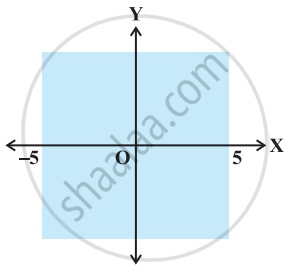
|x| < 5
|x| ≤ 5
|x| > 5
|x| ≥ 5
Objective the Questions from 27 to 30
Solution of a linear inequality in variable x is represented on number line given below ______.

x ∈ (–∞, 5)
x ∈ (–∞, 5]
x ∈ [5, ∞)
x ∈ (5, ∞)
Solution of a linear inequality in variable x is represented on number line given below ______.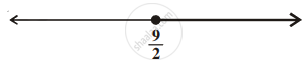
x ∈
x ∈
x ∈
x ∈
Solution of a linear inequality in variable x is represented on number line given below ______.
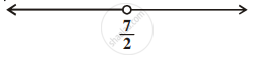
x ∈
x ∈
x ∈
x ∈
Solution of a linear inequality in variable x is represented on number line given below ______.
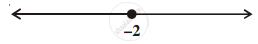
x ∈ (–∞, –2)
x ∈ (–∞, –2]
x ∈ (–2, ∞]
x ∈ [–2, ∞)
State whether the following statement is True or False:
State which of the following statement is True or False.
If x < y and b < 0, then
True
False
State which of the following statement is True or False.
If xy > 0, then x > 0 and y < 0
True
False
State which of the following statement is True or False.
If xy > 0, then x < 0 and y < 0
True
False
State which of the following statement is True or False.
If xy < 0, then x < 0 and y < 0
True
False
State which of the following statement is True or False.
If x < –5 and x < –2, then x ∈ (–∞, –5)
True
False
If x < –5 and x > 2, then x ∈ (– 5, 2)
True
False
If x > –2 and x < 9, then x ∈ (– 2, 9)
True
False
If |x| > 5, then x ∈ (–
True
False
If |x| ≤ 4, then x ∈ [– 4, 4]
True
False
Graph of x < 3 is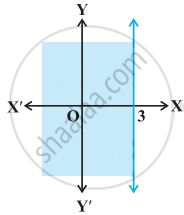
True
False
Graph of x ≥ 0 is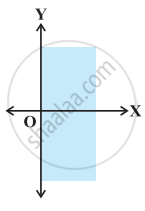
True
False
Graph of y ≤ 0 is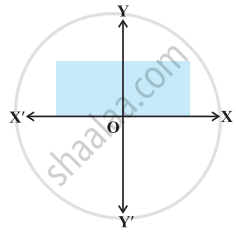
True
False
Solution set of x ≥ 0 and y ≤ 0 is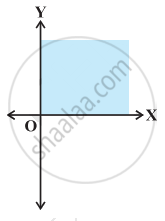
True
False
Solution set of x ≥ 0 and y ≤ 1 is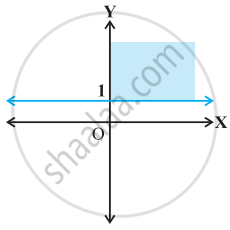
True
False
Solution set of x + y ≥ 0 is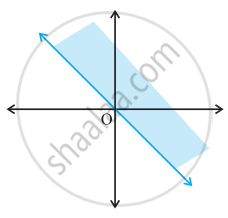
True
False
Fill in the blanks of the following:
If – 4x ≥ 12, then x ______ – 3.
If
If
If x > – 5, then 4x ______ –20.
If x > y and z < 0, then – xz ______ – yz.
If p > 0 and q < 0, then p – q ______ p.
If |x + 2| > 5, then x ______ – 7 or x ______ 3.
If – 2x + 1 ≥ 9, then x ______ – 4.
Solutions for 6: Linear Inequalities
![NCERT Exemplar solutions for Mathematics [English] Class 11 chapter 6 - Linear Inequalities NCERT Exemplar solutions for Mathematics [English] Class 11 chapter 6 - Linear Inequalities - Shaalaa.com](/images/mathematics-english-class-11_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
NCERT Exemplar solutions for Mathematics [English] Class 11 chapter 6 - Linear Inequalities
Shaalaa.com has the CBSE Mathematics Mathematics [English] Class 11 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. NCERT Exemplar solutions for Mathematics Mathematics [English] Class 11 CBSE 6 (Linear Inequalities) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. NCERT Exemplar textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 11 chapter 6 Linear Inequalities are Inequalities - Introduction, Algebraic Solutions of Linear Inequalities in One Variable and Their Graphical Representation, Graphical Solution of Linear Inequalities in Two Variables, Solution of System of Linear Inequalities in Two Variables.
Using NCERT Exemplar Mathematics [English] Class 11 solutions Linear Inequalities exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in NCERT Exemplar Solutions are essential questions that can be asked in the final exam. Maximum CBSE Mathematics [English] Class 11 students prefer NCERT Exemplar Textbook Solutions to score more in exams.
Get the free view of Chapter 6, Linear Inequalities Mathematics [English] Class 11 additional questions for Mathematics Mathematics [English] Class 11 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.
