Advertisements
Advertisements
Question
The center O of a circle of a radius 1.3 cm is at a distance of 3.8 cm from a given straight line AB. Draw a circle to touch the given straight line AB at a point P so that OP = 4.7 cm and to touch the given circle externally.
Solution
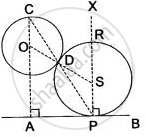
Steps of construction:
1) Take a point O at a distance of 3.8 cm from AB and with O as centre draw a circle of radius 1.3 cm.
2) With O as centre and radius equal to 4.7 cm, draw an arc cut BA at P. Draw PX perpendicular to AB.
3) Produce AO to cut the circle at C and join CP cutting the circle at D.
4) Join OD and produce it to cut PX at S. With S as centre and radius = SD, draw the circle PDR. This is the required circle.
APPEARS IN
RELATED QUESTIONS
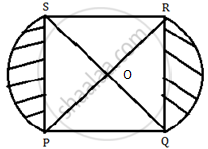
In the figure given below, PQRS is square lawn with side PQ = 42 metres. Two circular flower beds are there on the sides PS and QR with centre at O, the intersections of its
diagonals. Find the total area of the two flower beds (shaded parts).
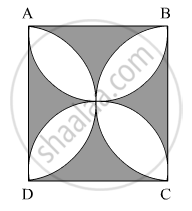
In Fig. 4, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
(use `pi=22/7`)
Find the area of the sector of a circle whose radius is 14 cm and angle of sector is 45º
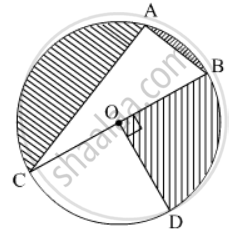
In the given figure, O is the centre of the circle with AC = 24 cm, AB = 7 cm and ∠BOD = 90°. Find the area of the shaded region.
Find the area of trapezium whose parallel sides are 11 m and 25 m long, and the nonparallel sides are 15 m and 13 m long.
Find the circumferences of a circle whose radius is 7 cm.
The circumference of a circle is 22 cm. Find the area of its quadrant.
Find the area of a quadrant of a circle whose circumference is 88 cm.
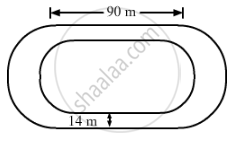
The inside perimeter of a running track shown in the figure is 400 m. The length of each of the straight portions is 90 m, and the ends are semicircles. If the track is 14 m wide everywhere, find the area of the track. Also, find the length of the outer boundary of the track.
The area of the circle is given below; find the diameter.
176 sq cm
The diameter of a cycle wheel is `4 5/11` cm. How many revolution will it make in moving 6.3 km ?
Find the curved surface area , the total surface area and the volume of a cone if its :
Height = 8 cm , diameter = 12 cm
A hollow metallic cylindrical tube has an internal radius of 3.5 cm and height 21 cm. The thickness of the metal tube is 0.5 cm. The tube is melted and cast into a right circular cone of height 7 cm. Find the radius of the cone, correct to one decimal place.
A wheel has a diameter of 84 cm. Find how many completer revolutions must it make to cover 3.168 km.
In the adjoining figure, the crescent is formed by two circles which touch at the point A. O is the centre of bigger circle. If CB = 9 cm and DE = 5 cm, find the area of the shaded portion.
Find the area and perimeter of the following semicircles: Diameter = 5.6cm
The sum of the circumference and diameter of a circle is 176 cm. Find the area of the circle.
Diameters of different circles are given below. Find their circumference (Take π = `22/7`)
d = 56 m
Areas of two circles are equal. Is it necessary that their circumferences are equal? Why?
Ratio of circumference of a circle to its radius is always 2π:I.
