Advertisements
Advertisements
Question
The line-width of a bright fringe is sometimes defined as the separation between the points on the two sides of the central line where the intensity falls to half the maximum. Find the line-width of a bright fringe in a Young's double slit experiment in terms of \[\lambda,\] d and D where the symbols have their usual meanings.
Solution
Given:-
Separation between two slits = d
Wavelength of the light = \[\lambda\]
Distance of the screen = D
Let Imax be the maximum intensity and I be half the maximum intensity at a point at a distance y from the central point.
So, \[I = a^2 + a^2 + 2 a^2 \cos\phi\]
Here, \[\phi\] is the phase difference in the waves coming from the two slits.
So, \[I = 4 a^2 \cos^2 \left( \frac{\phi}{2} \right)\]
\[\Rightarrow \frac{I}{I_\max} = \frac{1}{2}\]
\[ \Rightarrow \frac{4 a^2 \cos^2 \left( \frac{\phi}{2} \right)}{4 a^2} = \frac{1}{2}\]
\[ \Rightarrow \cos^2 \left( \frac{\phi}{2} \right) = \frac{1}{2}\]
\[ \Rightarrow \cos\left( \frac{\phi}{2} \right) = \frac{1}{\sqrt{2}}\]
\[ \Rightarrow \frac{\phi}{2} = \frac{\pi}{4}\]
\[ \Rightarrow \phi = \frac{\pi}{2}\]
Corrosponding path difference, \[∆ x = \frac{1}{4}\]
\[ \Rightarrow y = \frac{∆ xD}{d} = \frac{\lambda D}{4d}\]
The line-width of a bright fringe is defined as the separation between the points on the two sides of the central line where the intensity falls to half the maximum.
So, line-width = 2y
\[= 2\frac{D\lambda}{4d} = \frac{D\lambda}{2d}\]
Thus, the required line width of the bright fringe is \[\frac{D\lambda}{2d}.\]
APPEARS IN
RELATED QUESTIONS
(i) In Young's double-slit experiment, deduce the condition for (a) constructive and (b) destructive interferences at a point on the screen. Draw a graph showing variation of intensity in the interference pattern against position 'x' on the screen.
(b) Compare the interference pattern observed in Young's double-slit experiment with single-slit diffraction pattern, pointing out three distinguishing features.
In young’s double slit experiment, deduce the conditions for obtaining constructive and destructive interference fringes. Hence, deduce the expression for the fringe width.
Show that the angular width of the first diffraction fringe is half that of the central fringe.
The ratio of the intensities at minima to the maxima in the Young's double slit experiment is 9 : 25. Find the ratio of the widths of the two slits.
In a Young’s double-slit experiment, the slits are separated by 0.28 mm and the screen is placed 1.4 m away. The distance between the central bright fringe and the fourth bright fringe is measured to be 1.2 cm. Determine the wavelength of light used in the experiment.
Using analytical method for interference bands, obtain an expression for path difference between two light waves.
The fringes produced in diffraction pattern are of _______.
(A) equal width with same intensity
(B) unequal width with varying intensity
(C) equal intensity\
(D) equal width with varying intensity
If the source of light used in a Young's double slit experiment is changed from red to violet, ___________ .
White light is used in a Young's double slit experiment. Find the minimum order of the violet fringe \[\left( \lambda = 400\text{ nm} \right)\] which overlaps with a red fringe \[\left( \lambda = 700\text{ nm} \right).\]
A mica strip and a polystyrene strip are fitted on the two slits of a double slit apparatus. The thickness of the strips is 0.50 mm and the separation between the slits is 0.12 cm. The refractive index of mica and polystyrene are 1.58 and 1.55, respectively, for the light of wavelength 590 nm which is used in the experiment. The interference is observed on a screen at a distance one metre away. (a) What would be the fringe-width? (b) At what distance from the centre will the first maximum be located?
A thin paper of thickness 0.02 mm having a refractive index 1.45 is pasted across one of the slits in a Young's double slit experiment. The paper transmits 4/9 of the light energy falling on it. (a) Find the ratio of the maximum intensity to the minimum intensity in the fringe pattern. (b) How many fringes will cross through the centre if an identical paper piece is pasted on the other slit also? The wavelength of the light used is 600 nm.
In a Young's double slit experiment, \[\lambda = 500\text{ nm, d = 1.0 mm and D = 1.0 m.}\] Find the minimum distance from the central maximum for which the intensity is half of the maximum intensity.
In Young's double slit experiment using monochromatic light of wavelength 600 nm, 5th bright fringe is at a distance of 0·48 mm from the centre of the pattern. If the screen is at a distance of 80 cm from the plane of the two slits, calculate:
(i) Distance between the two slits.
(ii) Fringe width, i.e. fringe separation.
Two balls are projected at an angle θ and (90° − θ) to the horizontal with the same speed. The ratio of their maximum vertical heights is:
Consider a two-slit interference arrangement (Figure) such that the distance of the screen from the slits is half the distance between the slits. Obtain the value of D in terms of λ such that the first minima on the screen falls at a distance D from the centre O.
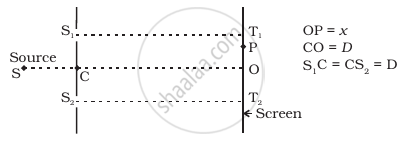
ASSERTION (A): In an interference pattern observed in Young's double slit experiment, if the separation (d) between coherent sources as well as the distance (D) of the screen from the coherent sources both are reduced to 1/3rd, then new fringe width remains the same.
REASON (R): Fringe width is proportional to (d/D).
How will the interference pattern in Young's double-slit experiment be affected if the source slit is moved away from the plane of the slits?
A beam of light consisting of two wavelengths 600 nm and 500 nm is used in Young's double slit experiment. The silt separation is 1.0 mm and the screen is kept 0.60 m away from the plane of the slits. Calculate:
- the distance of the second bright fringe from the central maximum for wavelength 500 nm, and
- the least distance from the central maximum where the bright fringes due to both wavelengths coincide.
Monochromatic green light of wavelength 5 × 10-7 m illuminates a pair of slits 1 mm apart. The separation of bright lines in the interference pattern formed on a screen 2 m away is ______.
In an interference experiment, a third bright fringe is obtained at a point on the screen with a light of 700 nm. What should be the wavelength of the light source in order to obtain the fifth bright fringe at the same point?
