Advertisements
Advertisements
Question
The median of an equilateral triangle is increasing at the ratio of `2sqrt(3)` cm/s. Find the rate at which its side is increasing.
Advertisements
Solution
Let ‘a’ be the side of an equilateral triangle.
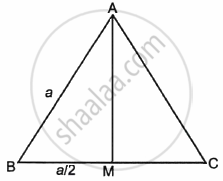
Medain AM = `sqrt(3)/2a`
Given `(d(AM))/(dt) = 2sqrt(3)` cm/s
`(d(AM))/(dt) = (d(sqrt(3)/2a))/(da) xx (da)/dt`
`2sqrt(3) = sqrt(3)/2 . (da)/dt`
`\implies (da)/dt` = 4 cm/s
APPEARS IN
RELATED QUESTIONS
The Volume of cube is increasing at the rate of 9 cm 3/s. How fast is its surfacee area increasing when the length of an edge is 10 cm?
The length x of a rectangle is decreasing at the rate of 5 cm/minute and the width y is increasing at the rate of 4 cm/minute. When x = 8 cm and y = 6 cm, find the rates of change of (a) the perimeter, and (b) the area of the rectangle.
Sand is pouring from a pipe at the rate of 12 cm3/s. The falling sand forms a cone on the ground in such a way that the height of the cone is always one-sixth of the radius of the base. How fast is the height of the sand cone increasing when the height is 4 cm?
The total revenue in rupees received from the sale of x units of a product is given by R(x) = 3x2 + 36x + 5. The marginal revenue, when x = 15 is ______.
The volume of a sphere is increasing at the rate of 3 cubic centimeter per second. Find the rate of increase of its surface area, when the radius is 2 cm
Find the rate of change of the volume of a cone with respect to the radius of its base ?
The side of a square sheet is increasing at the rate of 4 cm per minute. At what rate is the area increasing when the side is 8 cm long?
The radius of a circle is increasing at the rate of 0.7 cm/sec. What is the rate of increase of its circumference?
A balloon which always remains spherical, is being inflated by pumping in 900 cubic centimetres of gas per second. Find the rate at which the radius of the balloon is increasing when the radius is 15 cm.
A balloon in the form of a right circular cone surmounted by a hemisphere, having a diameter equal to the height of the cone, is being inflated. How fast is its volume changing with respect to its total height h, when h = 9 cm.
The surface area of a spherical bubble is increasing at the rate of 2 cm2/s. When the radius of the bubble is 6 cm, at what rate is the volume of the bubble increasing?
The volume of metal in a hollow sphere is constant. If the inner radius is increasing at the rate of 1 cm/sec, find the rate of increase of the outer radius when the radii are 4 cm and 8 cm respectively.
Sand is being poured onto a conical pile at the constant rate of 50 cm3/ minute such that the height of the cone is always one half of the radius of its base. How fast is the height of the pile increasing when the sand is 5 cm deep ?
A particle moves along the curve y = (2/3)x3 + 1. Find the points on the curve at which the y-coordinate is changing twice as fast as the x-coordinate ?
The volume of a spherical balloon is increasing at the rate of 25 cm3/sec. Find the rate of change of its surface area at the instant when radius is 5 cm ?
The length x of a rectangle is decreasing at the rate of 5 cm/minute and the width y is increasing at the rate of 4 cm/minute. When x = 8 cm and y = 6 cm, find the rates of change of the area of the rectangle.
Find the surface area of a sphere when its volume is changing at the same rate as its radius ?
A ladder, 5 metre long, standing on a horizontal floor, leans against a vertical wall. If the top of the ladder slides down wards at the rate of 10 cm/sec, then find the rate at which the angle between the floor and ladder is decreasing when lower end of ladder is 2 metres from the wall ?
The coordinates of the point on the ellipse 16x2 + 9y2 = 400 where the ordinate decreases at the same rate at which the abscissa increases, are
The radius of the base of a cone is increasing at the rate of 3 cm/minute and the altitude is decreasing at the rate of 4 cm/minute. The rate of change of lateral surface when the radius = 7 cm and altitude 24 cm is
The distance moved by a particle travelling in straight line in t seconds is given by s = 45t + 11t2 − t3. The time taken by the particle to come to rest is
If the rate of change of volume of a sphere is equal to the rate of change of its radius, then its radius is equal to
A man of height 6 ft walks at a uniform speed of 9 ft/sec from a lamp fixed at 15 ft height. The length of his shadow is increasing at the rate of
Evaluate: `int (x(1+x^2))/(1+x^4)dx`
The radius of a cylinder is increasing at the rate of 3 m/s and its height is decreasing at the rate of 4 m/s. The rate of change of volume when the radius is 4 m and height is 6 m, is ____________.
If the rate of change of the area of the circle is equal to the rate of change of its diameter then its radius is equal to ____________.
A man 1.6 m tall walks at the rate of 0.3 m/sec away from a street light that is 4 m above the ground. At what rate is the tip of his shadow moving? At what rate is his shadow lengthening?
A spherical balloon is filled with 4500π cubic meters of helium gas. If a leak in the balloon causes the gas to escape at the rate of 72π cubic meters per minute, then the rate (in meters per minute) at which the radius of the balloon decreases 49 minutes after the leakage began is ______.
