Advertisements
Advertisements
Question
The perimeter of a square plot of land is 64m. The area of a nearby rectangular plot is 24m2 more than the area of the given square. If the length of the rectangle is 14m, find its breadth.
Solution
The perimeter of a square with side s = P = 4s
∴ Here, the perimeter of the square 64m
Let the side of the square = s
∴ 4s = 64
⇒ s = 16m
We know, The area of a square with side s = s2
∴ s2
= 162
= 256m2
∴ The area of the rectangle
= 256 + 64
= 280m2
The area of a rectangle with length l and breadth b = A = l x b
Here, l = 14m, let breadth = b
The area of a rectangle with length 4 and breadth b = A = 14 x b
⇒ 14b = 280
⇒ b =
= 20m.
APPEARS IN
RELATED QUESTIONS
State, 'true' or 'false'
Every rhombus is a parallelogram.
State, 'true' or 'false'
Every parallelogram is a rhombus.
State, 'true' or 'false'
If the diagonals of a quadrilateral bisect each other at right angle, the quadrilateral is a square.
In the figure, given below, AM bisects angle A and DM bisects angle D of parallelogram ABCD. Prove that: ∠AMD = 90°.

In the given figure ABCD is a rhombus with angle A = 67°

If DEC is an equilateral triangle, calculate:
- ∠CBE
- ∠DBE
In a square ABCD, diagonals meet at O. P is a point on BC such that OB = BP.
Show that:
- ∠POC =
- ∠BDC = 2 ∠POC
- ∠BOP = 3 ∠CPO
In the given figure, PQRS is a ∥ gm. A straight line through P cuts SR at point T and QR produced at N. Prove that area of triangle QTR is equal to the area of triangle STN.
In the figure, ABCD is a parallelogram and APD is an equilateral triangle of side 80cm, Calculate the area of parallelogram ABCD.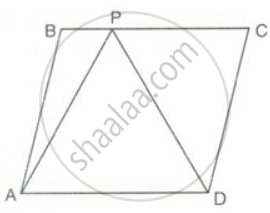
In the figure, PQR is a straight line. SQ is parallel to Tp. Prove that the quadrilateral PQST is equal in area to the ΔPSR.
Two adjacent sides of a parallelogram are 34 cm and 20 cm. If one of its diagonal is 42 cm, find: distance between its shorter sides
In a trapezium the parallel sides are 12cm and 8cm. If the distance between them is 6cm, find the area of the trapezium.
Find the perimeter and area of a square whose diagonal is
The side of a square exceeds the side of another square by 4cm and the sum of the areas of the squares is 400cm2. Find the dimensions of the squares.
Find the area of a rhombus whose perimeter is 260cm and the length of one of its diagonal is 66cm.
A rectangular field is 80m long and 50m wide. A 4m wide runs through the centre of the field parallel to the length and breadth of the field. Find the total area of the roads.
A lawn in the shape of a rectangle is to be developed in front of a Marriage Hall. The length and breadth of the lawn are 44m and 32m. A space of 2m is left on the two shoulder sides and one longer side for flower and in the remaining area grass is laid. Calculate the area of the flower space and the area on which grass is laid.
A rectangular field is 240m long and 180m broad. In one corner a farm house is built on a square plot of side 40m. Find the area of the remaining portion and the cost of fencing the open sides Rs.25per m.
Inside a square field of side 44m, a square flower bed is prepared leaving a graved path all round the flower bed. The total cost of laying the flower bed at Rs.25per sq m. and gravelling the path at Rs.120per sq m. is Rs.80320. Find the width of the gravel path.
Give reasons for the following :
A rectangle can be thought of as a special parallelogram.
Name polygon.

Make two more examples of this.
