Advertisements
Advertisements
Question
The probability distribution function of a random variable X is given by
| xi : | 0 | 1 | 2 |
| pi : | 3c3 | 4c − 10c2 | 5c-1 |
where c > 0 Find: c
Solution
We know that the sum of probabilities in a probability distribution is always 1.
∴ P (X = 0) + P (X = 1) + P (X = 2) = 1
\[\Rightarrow 3 c^3 + 4c - 10 c^2 + 5c - 1 = 1\]
\[ \Rightarrow 3 c^3 - 10 c^2 + 9c - 2 = 0\]
\[ \Rightarrow \left( c - 1 \right)\left( 3 c^2 - 7c + 2 \right) = 0\]
\[ \Rightarrow \left( c - 1 \right)\left( 3c - 1 \right)\left( c - 2 \right) = 0\]
\[ \Rightarrow c = \frac{1}{3}, 1, 2\]
\[\left( \text{ Neglecting 1 and 2 as individual probability should not be greater than one} \right)\]
APPEARS IN
RELATED QUESTIONS
An urn contains 5 red and 2 black balls. Two balls are randomly drawn. Let X represents the number of black balls. What are the possible values of X? Is X a random variable?
Assume that the chances of the patient having a heart attack are 40%. It is also assumed that a meditation and yoga course reduce the risk of heart attack by 30% and prescription of certain drug reduces its chances by 25%. At a time a patient can choose any one of the two options with equal probabilities. It is given that after going through one of the two options the patient selected at random suffers a heart attack. Find the probability that the patient followed a course of meditation and yoga?
A random variable X ~ N (0, 1). Find P(X > 0) and P(X < 0).
Two cards are drawn successively with replacement from well shuffled pack of 52 cards. Find the probability distribution of the number of aces.
Two cards are drawn successively without replacement from a well shuffled pack of 52 cards. Find the probability distribution of the number of aces.
Find the probability distribution of the number of white balls drawn in a random draw of 3 balls without replacement, from a bag containing 4 white and 6 red balls
Four balls are to be drawn without replacement from a box containing 8 red and 4 white balls. If X denotes the number of red balls drawn, then find the probability distribution of X.
Let, X denote the number of colleges where you will apply after your results and P(X = x) denotes your probability of getting admission in x number of colleges. It is given that
where k is a positive constant. Find the value of k. Also find the probability that you will get admission in (i) exactly one college (ii) at most 2 colleges (iii) at least 2 colleges.
Find the mean and standard deviation of each of the following probability distribution :
| xi : | -5 | -4 | 1 | 2 |
| pi : | \[\frac{1}{4}\] | \[\frac{1}{8}\] | \[\frac{1}{2}\] | \[\frac{1}{8}\] |
Find the mean and standard deviation of each of the following probability distribution :
| xi: | 0 | 1 | 3 | 5 |
| pi : | 0.2 | 0.5 | 0.2 | 0.1 |
Find the mean and standard deviation of each of the following probability distribution :
| xi : | -3 | -1 | 0 | 1 | 3 |
| pi : | 0.05 | 0.45 | 0.20 | 0.25 | 0.05 |
Find the mean and standard deviation of each of the following probability distribution :
| xi : | 0 | 1 | 2 | 3 | 4 | 5 |
| pi : |
\[\frac{1}{6}\]
|
\[\frac{5}{18}\]
|
\[\frac{2}{9}\]
|
\[\frac{1}{6}\]
|
\[\frac{1}{9}\]
|
\[\frac{1}{18}\]
|
A pair of fair dice is thrown. Let X be the random variable which denotes the minimum of the two numbers which appear. Find the probability distribution, mean and variance of X.
A fair die is tossed. Let X denote twice the number appearing. Find the probability distribution, mean and variance of X.
Two cards are selected at random from a box which contains five cards numbered 1, 1, 2, 2, and 3. Let X denote the sum and Y the maximum of the two numbers drawn. Find the probability distribution, mean and variance of X and Y.
In a game, a man wins Rs 5 for getting a number greater than 4 and loses Rs 1 otherwise, when a fair die is thrown. The man decided to thrown a die thrice but to quit as and when he gets a number greater than 4. Find the expected value of the amount he wins/loses.
If X denotes the number on the upper face of a cubical die when it is thrown, find the mean of X.
If the probability distribution of a random variable X is given by Write the value of k.
| X = xi : | 1 | 2 | 3 | 4 |
| P (X = xi) : | 2k | 4k | 3k | k |
A random variable X takes the values 0, 1, 2, 3 and its mean is 1.3. If P (X = 3) = 2 P (X = 1) and P (X = 2) = 0.3, then P (X = 0) is
A die is tossed twice. A 'success' is getting an even number on a toss. Find the variance of number of successes.
Three cards are drawn successively with replacement from a well shuffled pack of 52 cards. Find the probability distribution of the number of spades. Hence, find the mean of the distribtution.
Two fair coins are tossed simultaneously. If X denotes the number of heads, find the probability distribution of X. Also find E(X).
Compute the age specific death rate for the following data :
| Age group (years) | Population (in thousands) | Number of deaths |
| Below 5 | 15 | 360 |
| 5-30 | 20 | 400 |
| Above 30 | 10 | 280 |
The probability that a bomb dropped from an aeroplane will strike a target is `1/5`, If four bombs are dropped, find the probability that :
(a) exactly two will strike the target,
(b) at least one will strike the target.
Solve the following :
Identify the random variable as either discrete or continuous in each of the following. Write down the range of it.
20 white rats are available for an experiment. Twelve rats are male. Scientist randomly selects 5 rats number of female rats selected on a specific day
Solve the following:
Identify the random variable as either discrete or continuous in each of the following. Write down the range of it.
A highway safety group is interested in studying the speed (km/hrs) of a car at a check point.
Determine whether each of the following is a probability distribution. Give reasons for your answer.
| y | –1 | 0 | 1 |
| P(y) | 0.6 | 0.1 | 0.2 |
A sample of 4 bulbs is drawn at random with replacement from a lot of 30 bulbs which includes 6 defective bulbs. Find the probability distribution of the number of defective bulbs.
A die is thrown 4 times. If ‘getting an odd number’ is a success, find the probability of 2 successes
A die is thrown 4 times. If ‘getting an odd number’ is a success, find the probability of at most 2 successes.
Defects on plywood sheet occur at random with the average of one defect per 50 Sq.ft. Find the probability that such a sheet has no defect
State whether the following is True or False :
If r.v. X assumes the values 1, 2, 3, ……. 9 with equal probabilities, E(x) = 5.
Solve the following problem :
It is observed that it rains on 10 days out of 30 days. Find the probability that it rains on exactly 3 days of a week.
Find the mean and variance of the number randomly selected from 1 to 15
Consider the probability distribution of a random variable X:
| X | 0 | 1 | 2 | 3 | 4 |
| P(X) | 0.1 | 0.25 | 0.3 | 0.2 | 0.15 |
Variance of X.
A bag contains 1 red and 3 white balls. Find the probability distribution of the number of red balls if 2 balls are drawn at random from the bag one-by-one without replacement.
Two balls are drawn at random one by one with replacement from an urn containing equal number of red balls and green balls. Find the probability distribution of number of red balls. Also, find the mean of the random variable.
Kiran plays a game of throwing a fair die 3 times but to quit as and when she gets a six. Kiran gets +1 point for a six and –1 for any other number.
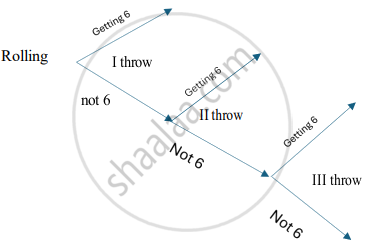
- If X denotes the random variable “points earned” then what are the possible values X can take?
- Find the probability distribution of this random variable X.
- Find the expected value of the points she gets.
