Advertisements
Advertisements
Question
The ratio in which the x-axis divides the line segment joining the points A (a1, b1) and B (a2, b2) is
Options
b1 : b2
−b1 : b2
a1 : a2
−a1 : a2
Solution
−b1 : b2
Explanation;
Hint:
A line divides internally in the ratio m : n the point P is, `(("m"x_2 + "n"x_1)/("m" + "n"), ("m"_2 +"n"y_1)/("m" + "n"))`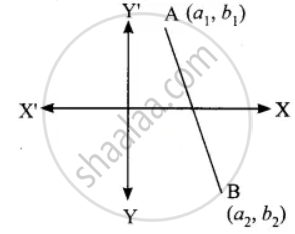
The point P is (a, 0) = `(("ma"_2 + "na"_1)/("m" + "n"), ("mb"_2 + "nb"_1)/("m" "n"))`
∴ `("mb"_2 + "nb"_1)/("m" + "n")` = 0
mb2 + nb1 = 0
⇒ mb2 = −nb1
`"m"/"n" = "b"_1/"b"_2`
∴ m : n = −b1 : b2
APPEARS IN
RELATED QUESTIONS
The co-ordinates of the centroid of a triangle PQR are (2, –5). If Q = (–6, 5) and R = (11, 8); calculate the co-ordinates of vertex P.
The mid-point of the line segment joining (2a, 4) and (–2, 2b) is (1, 2a + 1). Find the values of a and b.
Prove that the points A(–5, 4); B(–1, –2) and C(5, 2) are the vertices of an isosceles right-angled triangle. Find the co-ordinates of D so that ABCD is a square.
In the following example find the co-ordinate of point A which divides segment PQ in the ratio a : b.
P(–2, –5), Q(4, 3), a : b = 3 : 4
Write the co-ordinates of the point of intersection of graphs of
equations x = 2 and y = -3.
Complete the table below the graph with the help of the following graph.

| Sr. No. | First point | Second point | Co-ordinates of first point (x1 , y1) | Co-ordinates of second point (x2 , y2) | `(y_2 - y_2)/(x_2 - x_2)` |
| 1 | C | E | (1, 0) | (3,4) | `4/2=2` |
| 2 | A | B | (-1,-4) | (0,-2) | `2/1 = 2` |
| 3 | B | D | (0,-2) | (2,2) | `4/2=2` |
Find the centroid of a triangle whose vertices are (3, -5), (-7, 4) and ( 10, -2).
Find the mid-point of the line segment joining the points
(−2, 3) and (−6, −5)
If (1, −2), (3, 6), (x, 10) and (3, 2) are the vertices of the parallelogram taken in order, then the value of x is
Point M (2, b) is the mid-point of the line segment joining points P (a, 7) and Q (6, 5). Find the values of ‘a’ and ‘b’.
