SSC (English Medium)
SSC (Marathi Semi-English)
Academic Year: 2023-2024
Date & Time: 15th March 2024, 11:00 am
Duration: 2h
Advertisements
Note -
- All questions are compulsory.
- Use of a calculator is not allowed.
- The numbers to the right of the questions indicate full marks.
- In case of MCQs [Q. No. 1(A)] only the first attempt will be evaluated
and will be given credit. - Draw proper figures wherever necessary.
- The marks of construction should be clear. Do not erase them.
- Diagram is essential for writing the proof of the theorem.
Four alternative answers for the following question are given. Choose the correct alternative and write its alphabet:
Out of the dates given below which date constitutes a Pythagorean triplet?
15/8/17
16/8/16
3/5/17
4/9/15
Chapter: [0.02] Pythagoras Theorem
Four alternative answers for the following question are given. Choose the correct alternative and write its alphabet:
sin θ × cosec θ = ______
1
0
`1/2`
`sqrt2`
Chapter: [0.06] Trigonometry
Four alternative answers for the following question are given. Choose the correct alternative and write its alphabet:
Slope of X-axis is ______.
1
−1
0
Cannot be determined
Chapter: [0.05] Co-ordinate Geometry
Four alternative answers for the following question are given. Choose the correct alternative and write its alphabet:
A circle having radius 3 cm, then the length of its largest chord is ______.
1.5 cm
3 cm
6 cm
9 cm
Chapter: [0.03] Circle
If ΔABC ∼ ∆PQR and AB : PQ = 2 : 3, then find the value of `(A(triangleABC))/(A(trianglePQR))`.
Chapter: [0.01] Similarity
Two circles of radii 5 cm and 3 cm touch each other externally. Find the distance between their centres.
Chapter: [0.06] Trigonometry
Find the side of a square whose diagonal is `10sqrt2` cm.
Chapter: [0.02] Pythagoras Theorem
Angle made by the line with the positive direction of X-axis is given. Find the slope of the line.
45°
Chapter: [0.05] Co-ordinate Geometry
Advertisements

In the above figure, ∠ABC is inscribed in arc ABC.
If ∠ABC = 60°. find m ∠AOC.
Solution:
∠ABC = `1/2` m(arc AXC) ......`square`
60° = `1/2` m(arc AXC)
`square` = m(arc AXC)
But m ∠AOC = \[\boxed{m(arc ....)}\] ......(Property of central angle)
∴ m ∠AOC = `square`
Chapter: [0.03] Circle
Find the value of sin2θ + cos2θ

Solution:
In Δ ABC, ∠ABC = 90°, ∠C = θ°
AB2 + BC2 = `square` .....(Pythagoras theorem)
Divide both sides by AC2
`"AB"^2/"AC"^2 + "BC"^2/"AC"^2 = "AC"^2/"AC"^2`
∴ `("AB"^2/"AC"^2) + ("BC"^2/"AC"^2) = 1`
But `"AB"/"AC" = square and "BC"/"AC" = square`
∴ `sin^2 theta + cos^2 theta = square`
Chapter: [0.06] Trigonometry
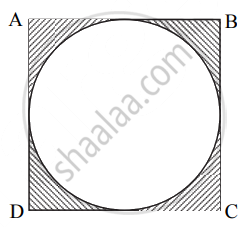
In the figure given above, `square`ABCD is a square and a circle is inscribed in it. All sides of a square touch the circle. If AB = 14 cm, find the area of shaded region.
Solution:
Area of square = `(square)^2` ......(Formula)
= 142
= `square "cm"^2`
Area of circle = `square` ......(Formula)
= `22/7 xx 7 xx 7`
= 154 cm2
(Area of shaded portion) = (Area of square) - (Area of circle)
= 196 − 154
= `square "cm"^2`
Chapter: [0.07] Mensuration
The radius of a sector of a circle is 3.5 cm and length of its arc is 2.2 cm. Find the area of the sector.
Chapter: [0.07] Mensuration
Find the length of the hypotenuse of a right angled triangle if remaining sides are 9 cm and 12 cm.
Chapter: [0.02] Pythagoras Theorem
In the given figure, m(arc NS) = 125°, m(arc EF) = 37°, find the measure ∠NMS.
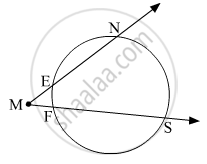
Chapter: [0.03] Circle
Find the slope of the line passing through the points A(2, 3) and B(4, 7).
Chapter: [0.05] Co-ordinate Geometry
Find the surface area of a sphere of radius 7 cm.
Chapter: [0.07] Mensuration
Advertisements

In ΔABC, ray BD bisects ∠ABC, A – D – C, seg DE || side BC, A – E – B, then for showing `("AB")/("BC") = ("AE")/("EB")`, complete the following activity:
Proof :
In ΔABC, ray BD bisects ∠B.
∴ `square/("BC") = ("AD")/("DC")` ...(I) (`square`)
ΔABC, DE || BC
∴ `(square)/("EB") = ("AD")/("DC")` ...(II) (`square`)
∴ `("AB")/square = square/("EB")` ...[from (I) and (II)]
Chapter: [0.01] Similarity
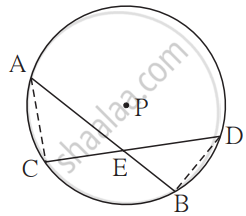
Given:
Chords AB and CD of a circle with centre P intersect at point E.
To prove:
AE × EB = CE × ED
Construction:
Draw seg AC and seg BD.
Fill in the blanks and complete the proof.
Proof:
In Δ CAE and Δ BDE,
∠AEC ≅ ∠DEB ...`square`
`square` ≅ ∠BDE ...(angles inscribed in the same arc)
∴ ΔCAE ~ ΔBDE ...`square`
∴ `square/ ("DE") = ("CE")/square` ...`square`
∴ AE × EB = CE × ED.
Chapter: [0.03] Circle
Determine whether the points are collinear.
A(1, −3), B(2, −5), C(−4, 7)
Chapter: [0.05] Co-ordinate Geometry
∆ABC ~ ∆LMN. In ∆ABC, AB = 5.5 cm, BC = 6 cm, CA = 4.5 cm. Construct ∆ABC and ∆LMN such that `"BC"/"MN" = 5/4`.
Chapter: [0.04] Geometric Constructions
In ΔPQR, seg PM is a median, PM = 9 and PQ2 + PR2 = 290. Find the length of QR.
Chapter: [0.02] Pythagoras Theorem
Prove that, if a line parallel to a side of a triangle intersects the other sides in two district points, then the line divides those sides in proportion.
Chapter: [0.01] Similarity
`1/sin^2θ - 1/cos^2θ - 1/tan^2θ - 1/cot^2θ - 1/sec^2θ - 1/("cosec"^2θ) = -3`, then find the value of θ.
Chapter: [0.06] Trigonometry
A cylinder of radius 12 cm contains water up to the height 20 cm. A spherical iron ball is dropped into the cylinder and thus water level raised by 6.75 cm. What is the radius of iron ball?
Chapter: [0.07] Mensuration
Draw a circle with centre O having radius 3 cm. Draw tangent segments PA and PB through the point P outside the circle such that ∠APB = 70°.
Chapter: [0.03] Circle
`square`ABCD is trapezium, AB || CD diagonals of trapezium intersects in point P.
Write the answers of the following questions:
- Draw the figure using the given information.
- Write any one pair of alternate angles and opposite angles.
- Write the names of similar triangles with the test of similarity.
Chapter: [0.01] Similarity
AB is a chord of a circle with centre O. AOC is diameter of circle, AT is a tangent at A.
Write answers of the following questions:
- Draw the figure using the given information.
- Find the measures of ∠CAT and ∠ABC with reasons.
- Whether ∠CAT and ∠ABC are congruent? Justify your answer.
Chapter: [0.03] Circle
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
Maharashtra State Board previous year question papers 10th Standard Board Exam Geometry Mathematics 2 with solutions 2023 - 2024
Previous year Question paper for Maharashtra State Board 10th Standard Board Exam Geometry Maths 2-2024 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Geometry Mathematics 2, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of Maharashtra State Board 10th Standard Board Exam.
How Maharashtra State Board 10th Standard Board Exam Question Paper solutions Help Students ?
• Question paper solutions for Geometry Mathematics 2 will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
