Advertisements
Advertisements
Question
Find the length of the hypotenuse of a right angled triangle if remaining sides are 9 cm and 12 cm.
Solution
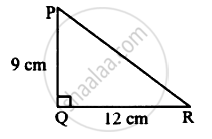
Let ∆PQR be the given right-angled triangle.
In ∆PQR, ∠Q = 90°
∴ PR2 = PQ2 + QR2 .......[Pythagoras theorem]
∴ PR2 = 92 + 122
∴ PR2 = 81 + 144
∴ PR2 = 225
∴ PR = `sqrt(225)` .....[Taking the square root of both sides]
∴ PR = 15 cm
∴ The length of the hypotenuse of the right-angled triangle is 15 cm.
RELATED QUESTIONS
A ladder leaning against a wall makes an angle of 60° with the horizontal. If the foot of the ladder is 2.5 m away from the wall, find the length of the ladder
In Fig., ∆ABC is an obtuse triangle, obtuse angled at B. If AD ⊥ CB, prove that AC2 = AB2 + BC2 + 2BC × BD
The perpendicular AD on the base BC of a ∆ABC intersects BC at D so that DB = 3 CD. Prove that `2"AB"^2 = 2"AC"^2 + "BC"^2`
ABC is an isosceles triangle with AC = BC. If AB2 = 2AC2, prove that ABC is a right triangle.
Two poles of heights 6 m and 11 m stand on a plane ground. If the distance between the feet of the poles is 12 m, find the distance between their tops.
Walls of two buildings on either side of a street are parallel to each other. A ladder 5.8 m long is placed on the street such that its top just reaches the window of a building at the height of 4 m. On turning the ladder over to the other side of the street, its top touches the window of the other building at a height 4.2 m. Find the width of the street.
In triangle ABC, AB = AC and BD is perpendicular to AC.
Prove that: BD2 - CD2 = 2CD × AD
In the following figure, OP, OQ, and OR are drawn perpendiculars to the sides BC, CA and AB respectively of triangle ABC.
Prove that: AR2 + BP2 + CQ2 = AQ2 + CP2 + BR2

The length of the diagonals of rhombus are 24cm and 10cm. Find each side of the rhombus.
Each side of rhombus is 10cm. If one of its diagonals is 16cm, find the length of the other diagonals.
In a triangle ABC, AC > AB, D is the midpoint BC, and AE ⊥ BC. Prove that: AC2 - AB2 = 2BC x ED
AD is perpendicular to the side BC of an equilateral ΔABC. Prove that 4AD2 = 3AB2.
In a right angled triangle, the hypotenuse is the greatest side
Find the distance between the helicopter and the ship
In the figure, find AR
Choose the correct alternative:
If length of sides of a triangle are a, b, c and a2 + b2 = c2, then which type of triangle it is?
Prove that the area of the semicircle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the semicircles drawn on the other two sides of the triangle.
In the adjoining figure, a tangent is drawn to a circle of radius 4 cm and centre C, at the point S. Find the length of the tangent ST, if CT = 10 cm.

In a right-angled triangle ABC, if angle B = 90°, then which of the following is true?
Two angles are said to be ______, if they have equal measures.
