Advertisements
Advertisements
प्रश्न
A committee of 7 has to be formed from 9 boys and 4 girls. In how many ways can this be done when the committee consists of:
(i) exactly 3 girls?
(ii) atleast 3 girls?
(iii) atmost 3 girls?
उत्तर
A committee of 7 has to be formed from 9 boys and 4 girls.
i) Since exactly 3 girls are to be there in every committee, each committee must consist of (7 – 3) = 4 boys only.
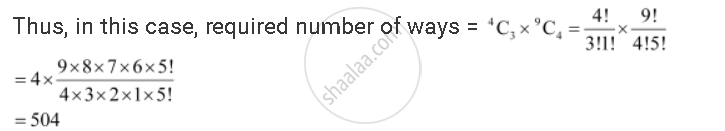
(ii) Since at least 3 girls are to be there in every committee, the committee can consist of
(a) 3 girls and 4 boys or (b) 4 girls and 3 boys
3 girls and 4 boys can be selected in `""^4C_3 xx ""^9C_4` ways.
4 girls and 3 boys can be selected in `""^4C_4 xx ""^9C_3` ways.

(iii) Since atmost 3 girls are to be there in every committee, the committee can consist of
(a) 3 girls and 4 boys (b) 2 girls and 5 boys
(c) 1 girl and 6 boys (d) No girl and 7 boys

APPEARS IN
संबंधित प्रश्न
In how many ways can a team of 3 boys and 3 girls be selected from 5 boys and 4 girls?
How many 6-digit numbers can be formed from the digits 0, 1, 3, 5, 7 and 9 which are divisible by 10 and no digit is repeated?
In a class there are 27 boys and 14 girls. The teacher wants to select 1 boy and 1 girl to represent the class in a function. In how many ways can the teacher make this selection?
A person wants to buy one fountain pen, one ball pen and one pencil from a stationery shop. If there are 10 fountain pen varieties, 12 ball pen varieties and 5 pencil varieties, in how many ways can he select these articles?
From Goa to Bombay there are two roots; air, and sea. From Bombay to Delhi there are three routes; air, rail and road. From Goa to Delhi via Bombay, how many kinds of routes are there?
A mint prepares metallic calendars specifying months, dates and days in the form of monthly sheets (one plate for each month). How many types of calendars should it prepare to serve for all the possibilities in future years?
Given 7 flags of different colours, how many different signals can be generated if a signal requires the use of two flags, one below the other?
A team consists of 6 boys and 4 girls and other has 5 boys and 3 girls. How many single matches can be arranged between the two teams when a boy plays against a boy and a girl plays against a girl?
How many three-digit numbers are there with no digit repeated?
In how many ways can six persons be seated in a row?
How many odd numbers less than 1000 can be formed by using the digits 0, 3, 5, 7 when repetition of digits is not allowed?
Evaluate the following:
14C3
f 24Cx = 24C2x + 3, find x.
If 15Cr : 15Cr − 1 = 11 : 5, find r.
In how many ways can a student choose 5 courses out of 9 courses if 2 courses are compulsory for every student?
There are 10 professors and 20 students out of whom a committee of 2 professors and 3 students is to be formed. Find the number of ways in which this can be done. Further find in how many of these committees:
a particular student is excluded.
From 4 officers and 8 jawans in how many ways can 6 be chosen (i) to include exactly one officer
In an examination, a student has to answer 4 questions out of 5 questions; questions 1 and 2 are however compulsory. Determine the number of ways in which the student can make the choice.
In how many ways can a committee of 5 persons be formed out of 6 men and 4 women when at least one woman has to be necessarily selected?
A group consists of 4 girls and 7 boys. In how many ways can a team of 5 members be selected if the team has (ii) at least one boy and one girl?
A group consists of 4 girls and 7 boys. In how many ways can a team of 5 members be selected if the team has(iii) at least 3 girls?
In an examination, a question paper consists of 12 questions divided into two parts i.e., Part I and Part II, containing 5 and 7 questions, respectively. A student is required to attempt 8 questions in all, selecting at least 3 from each part. In how many ways can a student select the questions?
If 15C3r = 15Cr + 3 , then r is equal to
Three persons enter a railway compartment. If there are 5 seats vacant, in how many ways can they take these seats?
The number of ways in which a host lady can invite for a party of 8 out of 12 people of whom two do not want to attend the party together is
The number of diagonals that can be drawn by joining the vertices of an octagon is
If n + 1C3 = 2 · nC2 , then n =
Find n if `""^6"P"_2 = "n" ""^6"C"_2`
Ten students are to be selected for a project from a class of 30 students. There are 4 students who want to be together either in the project or not in the project. Find the number of possible selections.
Find the value of 80C2
Find the value of 20C16 – 19C16
Answer the following:
A question paper has 6 questions. How many ways does a student have to answer if he wants to solve at least one question?
In how many ways can the letters of the word 'IMAGE' be arranged so that the vowels should always occupy odd places?
All the letters of the word ‘EAMCOT’ are arranged in different possible ways. The number of such arrangements in which no two vowels are adjacent to each other is ______.
A scientific committee is to be formed from 6 Indians and 8 foreigners, which includes at least 2 Indians and double the number of foreigners as Indians. Then the number of ways, the committee can be formed is ______.
All possible numbers are formed using the digits 1, 1, 2, 2, 2, 2, 3, 4, 4 taken all at a time. The number of such numbers in which the odd digits occupy even places is ______.
The number of positive integers satisfying the inequality `""^(n+1)C_(n-2) - ""^(n+1)C_(n-1) ≤ 100` is ______.
From 6 different novels and 3 different dictionaries, 4 novels and 1 dictionary are to be selected and arranged in a row on the shelf so that the dictionary is always in the middle. Then, the number of such arrangements is ______.
