Advertisements
Advertisements
प्रश्न
A committee of 7 has to be formed from 9 boys and 4 girls. In how many ways can this be done when the committee consists of:
(i) exactly 3 girls?
(ii) atleast 3 girls?
(iii) atmost 3 girls?
उत्तर
A committee of 7 has to be formed from 9 boys and 4 girls.
i) Since exactly 3 girls are to be there in every committee, each committee must consist of (7 – 3) = 4 boys only.
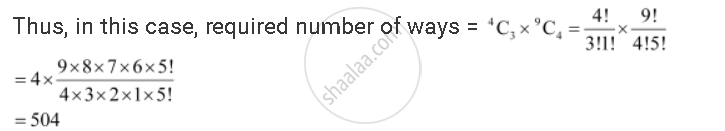
(ii) Since at least 3 girls are to be there in every committee, the committee can consist of
(a) 3 girls and 4 boys or (b) 4 girls and 3 boys
3 girls and 4 boys can be selected in `""^4C_3 xx ""^9C_4` ways.
4 girls and 3 boys can be selected in `""^4C_4 xx ""^9C_3` ways.

(iii) Since atmost 3 girls are to be there in every committee, the committee can consist of
(a) 3 girls and 4 boys (b) 2 girls and 5 boys
(c) 1 girl and 6 boys (d) No girl and 7 boys

APPEARS IN
संबंधित प्रश्न
Determine n if `""^(2n)C_3 : ""^nC_3 = 12 : 1`
Determine n if `""^(2n)C_3 : ""^nC_3 = 11: 1`
In how many ways can a team of 3 boys and 3 girls be selected from 5 boys and 4 girls?
In an examination, a question paper consists of 12 questions divided into two parts i.e., Part I and Part II, containing 5 and 7 questions, respectively. A student is required to attempt 8 questions in all, selecting at least 3 from each part. In how many ways can a student select the questions?
How many A.P.'s with 10 terms are there whose first term is in the set {1, 2, 3} and whose common difference is in the set {1, 2, 3, 4, 5}?
How many three-digit numbers are there with no digit repeated?
How many four-digit numbers can be formed with the digits 3, 5, 7, 8, 9 which are greater than 7000, if repetition of digits is not allowed?
How many four digit different numbers, greater than 5000 can be formed with the digits 1, 2, 5, 9, 0 when repetition of digits is not allowed?
Evaluate the following:
n + 1Cn
If 15C3r = 15Cr + 3, find r.
If 15Cr : 15Cr − 1 = 11 : 5, find r.
If 16Cr = 16Cr + 2, find rC4.
How many different selections of 4 books can be made from 10 different books, if
there is no restriction;
From 4 officers and 8 jawans in how many ways can 6 be chosen. to include at least one officer?
In a village, there are 87 families of which 52 families have at most 2 children. In a rural development programme, 20 families are to be helped chosen for assistance, of which at least 18 families must have at most 2 children. In how many ways can the choice be made?
A group consists of 4 girls and 7 boys. In how many ways can a team of 5 members be selected if the team has(iii) at least 3 girls?
Out of 18 points in a plane, no three are in the same straight line except five points which are collinear. How many (i) straight lines
How many different words, each containing 2 vowels and 3 consonants can be formed with 5 vowels and 17 consonants?
If 20Cr = 20Cr−10, then 18Cr is equal to
If nC12 = nC8 , then n =
If 43Cr − 6 = 43C3r + 1 , then the value of r is
Among 14 players, 5 are bowlers. In how many ways a team of 11 may be formed with at least 4 bowlers?
A lady gives a dinner party for six guests. The number of ways in which they may be selected from among ten friends if two of the friends will not attend the party together is
If n + 1C3 = 2 · nC2 , then n =
Find n if `""^6"P"_2 = "n" ""^6"C"_2`
Find n if `""^(2"n")"C"_3: ""^"n""C"_2` = 52:3
There are 8 doctors and 4 lawyers in a panel. Find the number of ways for selecting a team of 6 if at least one doctor must be in the team.
A student has to answer 10 questions, choosing atleast 4 from each of Parts A and B. If there are 6 questions in Part A and 7 in Part B, in how many ways can the student choose 10 questions?
A boy has 3 library tickets and 8 books of his interest in the library. Of these 8, he does not want to borrow Mathematics Part II, unless Mathematics Part I is also borrowed. In how many ways can he choose the three books to be borrowed?
The number of triangles that are formed by choosing the vertices from a set of 12 points, seven of which lie on the same line is ______.
The number of ways in which a team of eleven players can be selected from 22 players always including 2 of them and excluding 4 of them is ______.
A committee of 6 is to be chosen from 10 men and 7 women so as to contain atleast 3 men and 2 women. In how many different ways can this be done if two particular women refuse to serve on the same committee ______.
There are 12 points in a plane of which 5 points are collinear, then the number of lines obtained by joining these points in pairs is 12C2 – 5C2.
If some or all of n objects are taken at a time, the number of combinations is 2n – 1.
A candidate is required to answer 7 questions out of 12 questions which are divided into two groups, each containing 6 questions. He is not permitted to attempt more than 5 questions from either group. He can choose the seven questions in 650 ways.
To fill 12 vacancies there are 25 candidates of which 5 are from scheduled castes. If 3 of the vacancies are reserved for scheduled caste candidates while the rest are open to all, the number of ways in which the selection can be made is 5C3 × 20C9.
Number of selections of at least one letter from the letters of MATHEMATICS, is ______.
Total number of 6-digit numbers in which only and all the five digits 1, 3, 5, 7 and 9 appear is ______.
