Advertisements
Advertisements
рдкреНрд░рд╢реНрди
A point P is 26 cm away from O of circle and the length PT of the tangent drawn from P to the circle is 10 cm. Find the radius of the circle.
рдЙрддреНрддрд░
Given OP = 26 cm
PT = length of tangent = 10cm
radius = OT = ?
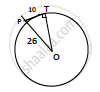
At point of contact, radius and tangent are perpendicular ∠OTP = 90°, ΔOTP is right
angled triangle.
By Pythagoras theorem, ЁЭСВЁЭСГ2 = ЁЭСВЁЭСЗ2 + ЁЭСГЁЭСЗ2
262 = ЁЭСВЁЭСЗ2 + 102
ЁЭСВЁЭСЗЁЭСШ =` (sqrt(676 − 100))`ЁЭСШ
ЁЭСВЁЭСЗ = `sqrt(576)`
= 24 cm
OT = length of tangent = 24 cm
APPEARS IN
рд╕рдВрдмрдВрдзрд┐рдд рдкреНрд░рд╢реНрди
In fig. a circle touches all the four sides of quadrilateral ABCD with AB = 6cm, BC = 7cm, CD = 4cm. Find AD.
O is the centre of a circle of radius 10 cm. P is any point in the circle such that OP = 6 cm. A is the point travelling along the circumference. x is the distance from A to P. what are the least and the greatest values of x in cm? what is the position of the points O, P and A at these values?
The length of three concesutive sides of a quadrilateral circumscribing a circle are 4 cm, 5 cm, and 7 cm respectively. Determine the length of the fourth side.
If all the sides of a parallelogram touch a circle, show that the parallelogram is a rhombus.
Use the figure given below to fill in the blank:
If PQ is 8 cm long, the length of RS = ________
State, if the following statement is true or false:
If the end points A and B of the line segment lie on the circumference of a circle, AB is a diameter.
If the radii of two concentric circles are 4 cm and 5 cm, then the length of each chord of one circle which is tangent to the other circle is ______
A point P is 10 cm from the center of a circle. The length of the tangent drawn from P to the circle is 8 cm. The radius of the circle is equal to ______
If a chord AB subtends an angle of 60° at the centre of a circle, then angle between the tangents at A and B is also 60°.
Prove that angle bisector of any angle of a triangle and perpendicular bisector of the opposite side if intersect, they will intersect on the circumcircle of the triangle.
