Advertisements
Advertisements
प्रश्न
AB is a chord of a circle with centre O. AOC is diameter of circle, AT is a tangent at A.
Write answers of the following questions:
- Draw the figure using the given information.
- Find the measures of ∠CAT and ∠ABC with reasons.
- Whether ∠CAT and ∠ABC are congruent? Justify your answer.
उत्तर

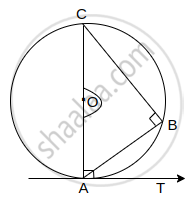
∠CAT = ∠OAT = 90° ...(By tangent theorem)
∠ABC = 90° ...(Angle in a semi-circle is right angle)- ∠CAT = ∠ABC
∠CAT ≅ ∠ABC ...[∴ The angle between a tangent of a circle and a chord drawn from the point of contact is congruent to the angle inscribed in the arc opposite to the arc intercepted by that angle.]
APPEARS IN
संबंधित प्रश्न
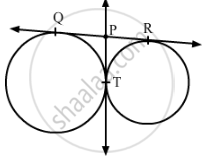
In Fig. 1, QR is a common tangent to the given circles, touching externally at the point T. The tangent at T meets QR at P. If PT = 3.8 cm, then the length of QR (in cm) is :
(A) 3.8
(B) 7.6
(C) 5.7
(D) 1.9
Points A(–1, y) and B(5, 7) lie on a circle with centre O(2, –3y). Find the values of y. Hence find the radius of the circle.
Fill in the blanks:
The longest chord of a circle is a __________ of the circle.
true or false
A chord of a circle, which is twice as long is its radius is a diameter of the circle.
In the given figure ABC is an isosceles triangle and O is the centre of its circumcircle. Prove that AP bisects angle BPC .

Find the length of tangent drawn to a circle with radius 8 cm form a point 17 cm away from the center of the circle
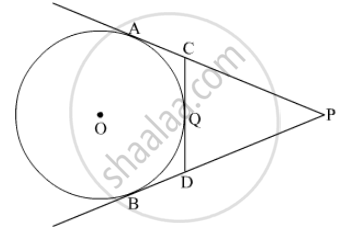
From an external point P, tangents PA and PB are drawn to a circle with center O. If CD is the tangent to the circle at a point E and PA = 14cm, find the perimeter of ΔPCD.

In the given figure, O is the centre of the circle. Find ∠CBD.

In the given figure, if ∠ABC = 45°, then ∠AOC =
In the given figure, PA and PB are tangents to the circle from an external point P. CD is another tangent touching the circle at Q. If PA = 12 cm, QC = QD = 3 cm, then find PC + PD.
Find the length of the chord of a circle in the following when:
Radius is 13 cm and the distance from the centre is 12 cm
Find the area of a circle of radius 7 cm.
In the given figure, seg MN is a chord of a circle with centre O. MN = 25, L is a point on chord MN such that ML = 9 and d(O,L) = 5. Find the radius of the circle.

Use the figure given below to fill in the blank:
R is the _______ of the circle.

Use the figure given below to fill in the blank:
______ is a chord of the circle.

State, if the following statement is true or false:
Every diameter bisects a circle and each part of the circle so obtained is a semi-circle.
Find the missing values in the following table for the circles with radius (r), diameter (d) and Circumference (C).
| radius (r) | diameter (d) | Circumference (C) |
| 15 cm |
Let s denote the semi-perimeter of a triangle ABC in which BC = a, CA = b, AB = c. If a circle touches the sides BC, CA, AB at D, E, F, respectively, prove that BD = s – b.
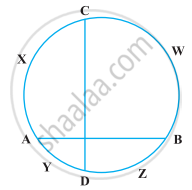
If two chords AB and CD of a circle AYDZBWCX intersect at right angles (see figure), prove that arc CXA + arc DZB = arc AYD + arc BWC = semi-circle.
In the given figure, O is the centre of the circle. Shade the smaller segment of the circle formed by CP.