Advertisements
Advertisements
प्रश्न
Find the area enclosed between the parabola 4y = 3x2 and the straight line 3x - 2y + 12 = 0.
उत्तर १
The given quations are
`y = (3x^2)/4` ....1
and, 3x – 2y + 12 = 0 ...(2)
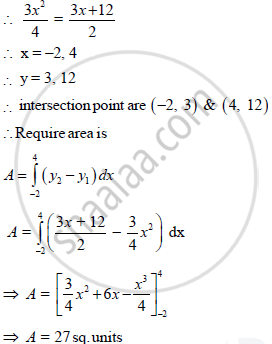
Solving equation no. (1) & (2)
y = `(3x + 12)/2`

उत्तर २
The equations of the curves are
4y = 3x2 .....(1)
3x – 2y + 12 = 0 .....(2)
The curve (1) represents a parabola having vertex at the origin, axis along the positive direction of y-axis and opens upwards.
The curve (2) represents a straight line. This straight line meets the x-axis at (−4, 0) and y-axis at (0, 6).
Soving (1) and (2), we get
`3x - 2((3x^2)/4) + 12 = 0`
⇒ 6x - 3x2 + 24 = 0
⇒ x2 - 2x−8=0
⇒ (x+2)(x−4) = 0
⇒ x = -2, 4
When x = − 2, y = 3
When x = 4, y = 12
So, the point of intersection of the given curves is (− 2, 3) and (4, 12).
The area bounded by the given curves is shown below.
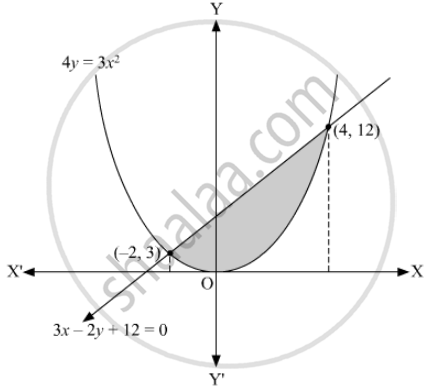
Required area = Area of the shaded region
= `int_(-2)^4 (y"Line" - y"Parabola") dx`
= `int_(-2)^4 ((3x + 12)/2 - 3/4 x^2) dx`
`= (3/4 x^2 + 6x - x^3/4)^4`
`= (3/4 xx 16 + 6 xx 4 - 64/4) - (3/4 xx 4 +6 xx (-2) + 3/4)`
= 20 - (- 7)
= 27 square units
संबंधित प्रश्न
Find the area under the given curve and given line:
y = x2, x = 1, x = 2 and x-axis
Find the area between the curves y = x and y = x2
Find the area of the smaller region bounded by the ellipse `x^2/a^2 + y^2/b^2 = 1` and the line `x/a + y/b = 1`
Using the method of integration find the area of the triangle ABC, coordinates of whose vertices are A(2, 0), B (4, 5) and C (6, 3).
Find the area of the region {(x, y) : y2 ≤ 4x, 4x2 + 4y2 ≤ 9}
Using the method of integration, find the area of the triangle ABC, coordinates of whose vertices are A (4 , 1), B (6, 6) and C (8, 4).
Find the area bounded by the circle x2 + y2 = 16 and the line `sqrt3 y = x` in the first quadrant, using integration.
Find the equation of an ellipse whose latus rectum is 8 and eccentricity is `1/3`
Find the area of the smaller region bounded by the ellipse \[\frac{x^2}{9} + \frac{y^2}{4} = 1\] and the line \[\frac{x}{3} + \frac{y}{2} = 1 .\]
Find the area of the region bounded by the parabola y2 = 16x and the line x = 4.
Find the area of the region bounded by the following curves, the X-axis and the given lines: y = `sqrt(16 - x^2)`, x = 0, x = 4
Choose the correct alternative :
Area of the region bounded by the curve x2 = y, the X-axis and the lines x = 1 and x = 3 is _______.
State whether the following is True or False :
The area bounded by the curve x = g (y), Y-axis and bounded between the lines y = c and y = d is given by `int_"c"^"d"x*dy = int_(y = "c")^(y = "d") "g"(y)*dy`
The area of the region lying in the first quadrant and bounded by the curve y = 4x2, and the lines y = 2 and y = 4 is ______
The area of the region bounded by y2 = 25x, x = 1 and x = 2 the X axis is ______
Find the area of the region bounded by the curve y = `sqrt(9 - x^2)`, X-axis and lines x = 0 and x = 3
Find the area of the region bounded by the curve y = `sqrt(2x + 3)`, the X axis and the lines x = 0 and x = 2
Find area of the region bounded by the parabola x2 = 4y, the Y-axis lying in the first quadrant and the lines y = 3
Find the area of the region bounded by the curve x = `sqrt(25 - y^2)`, the Y-axis lying in the first quadrant and the lines y = 0 and y = 5
Find the area of the circle x2 + y2 = 16
The area bounded by y = `27/x^3`, X-axis and the ordinates x = 1, x = 3 is ______
The area bounded by the X-axis, the curve y = f(x) and the lines x = 1, x = b is equal to `sqrt("b"^2 + 1) - sqrt(2)` for all b > 1, then f(x) is ______.
Equation of a common tangent to the circle, x2 + y2 – 6x = 0 and the parabola, y2 = 4x, is:
The area of the circle `x^2 + y^2 = 16`, exterior to the parabola `y = 6x`
The area of the region bounded by the curve y = x2, x = 0, x = 3, and the X-axis is ______.
The area (in sq.units) of the part of the circle x2 + y2 = 36, which is outside the parabola y2 = 9x, is ______.
Area in first quadrant bounded by y = 4x2, x = 0, y = 1 and y = 4 is ______.
The area bounded by the x-axis and the curve y = 4x – x2 – 3 is ______.
