Advertisements
Advertisements
प्रश्न
Find the coefficient of x5 in (x + 3)8
उत्तर
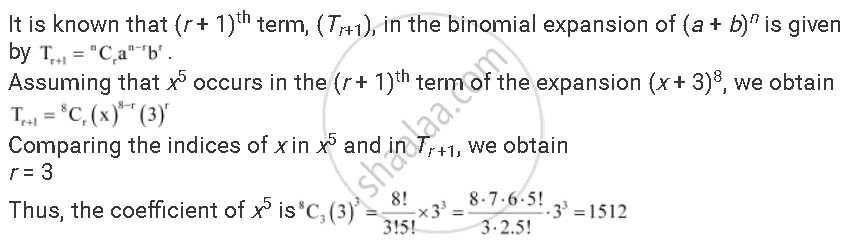
APPEARS IN
संबंधित प्रश्न
Write the general term in the expansion of (x2 – y)6
Find the 13th term in the expansion of `(9x - 1/(3sqrtx))^18 , x != 0`
Find the middle term in the expansion of:
(i) \[\left( \frac{2}{3}x - \frac{3}{2x} \right)^{20}\]
Find the middle terms in the expansion of:
(iii) \[\left( 3x - \frac{2}{x^2} \right)^{15}\]
Find the middle terms in the expansion of:
(iv) \[\left( x^4 - \frac{1}{x^3} \right)^{11}\]
Find the middle terms(s) in the expansion of:
(iii) \[\left( 1 + 3x + 3 x^2 + x^3 \right)^{2n}\]
Find the middle terms(s) in the expansion of:
(viii) \[\left( 2ax - \frac{b}{x^2} \right)^{12}\]
Find the term independent of x in the expansion of the expression:
(v) \[\left( \frac{\sqrt{x}}{3} + \frac{3}{2 x^2} \right)^{10}\]
Find the term independent of x in the expansion of the expression:
(vi) \[\left( x - \frac{1}{x^2} \right)^{3n}\]
Find the term independent of x in the expansion of the expression:
(ix) \[\left( \sqrt[3]{x} + \frac{1}{2 \sqrt[3]{x}} \right)^{18} , x > 0\]
Find a, if the coefficients of x2 and x3 in the expansion of (3 + ax)9 are equal.
Find the coefficient of a4 in the product (1 + 2a)4 (2 − a)5 using binomial theorem.
In the expansion of (1 + x)n the binomial coefficients of three consecutive terms are respectively 220, 495 and 792, find the value of n.
If the coefficients of three consecutive terms in the expansion of (1 + x)n be 76, 95 and 76, find n.
If the 2nd, 3rd and 4th terms in the expansion of (x + a)n are 240, 720 and 1080 respectively, find x, a, n.
Find a, b and n in the expansion of (a + b)n, if the first three terms in the expansion are 729, 7290 and 30375 respectively.
If p is a real number and if the middle term in the expansion of \[\left( \frac{p}{2} + 2 \right)^8\] is 1120, find p.
Write the coefficient of the middle term in the expansion of \[\left( 1 + x \right)^{2n}\] .
If in the expansion of (a + b)n and (a + b)n + 3, the ratio of the coefficients of second and third terms, and third and fourth terms respectively are equal, then n is
In the expansion of \[\left( x^2 - \frac{1}{3x} \right)^9\] , the term without x is equal to
The middle term in the expansion of \[\left( \frac{2 x^2}{3} + \frac{3}{2 x^2} \right)^{10}\] is
In the expansion of \[\left( x - \frac{1}{3 x^2} \right)^9\] , the term independent of x is
If in the expansion of (1 + y)n, the coefficients of 5th, 6th and 7th terms are in A.P., then nis equal to
If the sum of odd numbered terms and the sum of even numbered terms in the expansion of \[\left( x + a \right)^n\] are A and B respectively, then the value of \[\left( x^2 - a^2 \right)^n\] is
The middle term in the expansion of \[\left( \frac{2x}{3} - \frac{3}{2 x^2} \right)^{2n}\] is
Find the middle term in the expansion of `(2ax - b/x^2)^12`.
Find the term independent of x, x ≠ 0, in the expansion of `((3x^2)/2 - 1/(3x))^15`
Find the term independent of x in the expansion of `(3x - 2/x^2)^15`
Find the middle term (terms) in the expansion of `(x/a - a/x)^10`
Find the coefficient of `1/x^17` in the expansion of `(x^4 - 1/x^3)^15`
Find n in the binomial `(root(3)(2) + 1/(root(3)(3)))^n` if the ratio of 7th term from the beginning to the 7th term from the end is `1/6`
If xp occurs in the expansion of `(x^2 + 1/x)^(2n)`, prove that its coefficient is `(2n!)/(((4n - p)/3)!((2n + p)/3)!)`
In the expansion of `(x^2 - 1/x^2)^16`, the value of constant term is ______.
The sum of coefficients of the two middle terms in the expansion of (1 + x)2n–1 is equal to 2n–1Cn.
If the coefficient of x10 in the binomial expansion of `(sqrt(x)/5^(1/4) + sqrt(5)/x^(1/3))^60` is 5kl, where l, k ∈ N and l is coprime to 5, then k is equal to ______.
The sum of the real values of x for which the middle term in the binomial expansion of `(x^3/3 + 3/x)^8` equals 5670 is ______.
