Advertisements
Advertisements
प्रश्न
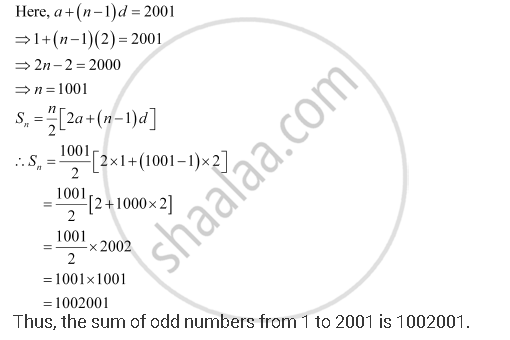
Find the sum of odd integers from 1 to 2001.
उत्तर
The odd integers from 1 to 2001 are 1, 3, 5, …1999, 2001.
This sequence forms an A.P.
Here, first term, a = 1
Common difference, d = 2
APPEARS IN
संबंधित प्रश्न
In an A.P, the first term is 2 and the sum of the first five terms is one-fourth of the next five terms. Show that 20th term is –112.
If the sum of n terms of an A.P. is 3n2 + 5n and its mth term is 164, find the value of m.
The sum of the first four terms of an A.P. is 56. The sum of the last four terms is 112. If its first term is 11, then find the number of terms.
A man deposited Rs 10000 in a bank at the rate of 5% simple interest annually. Find the amount in 15th year since he deposited the amount and also calculate the total amount after 20 years.
If the nth term an of a sequence is given by an = n2 − n + 1, write down its first five terms.
Let < an > be a sequence. Write the first five term in the following:
a1 = 1, an = an − 1 + 2, n ≥ 2
Let < an > be a sequence. Write the first five term in the following:
a1 = 1 = a2, an = an − 1 + an − 2, n > 2
The Fibonacci sequence is defined by a1 = 1 = a2, an = an − 1 + an − 2 for n > 2
Find `(""^an +1)/(""^an")` for n = 1, 2, 3, 4, 5.
Show that the following sequence is an A.P. Also find the common difference and write 3 more terms in case.
−1, 1/4, 3/2, 11/4, ...
Show that the following sequence is an A.P. Also find the common difference and write 3 more terms in case.
9, 7, 5, 3, ...
Find:
10th term of the A.P. 1, 4, 7, 10, ...
Which term of the A.P. 4, 9, 14, ... is 254?
Is 68 a term of the A.P. 7, 10, 13, ...?
If 9th term of an A.P. is zero, prove that its 29th term is double the 19th term.
Find the 12th term from the following arithmetic progression:
3, 8, 13, ..., 253
How many numbers of two digit are divisible by 3?
How many numbers are there between 1 and 1000 which when divided by 7 leave remainder 4?
If the sum of three numbers in A.P. is 24 and their product is 440, find the numbers.
Find the sum of first n natural numbers.
Find the sum of all integers between 84 and 719, which are multiples of 5.
Find the sum of all those integers between 100 and 800 each of which on division by 16 leaves the remainder 7.
Find the r th term of an A.P., the sum of whose first n terms is 3n2 + 2n.
Find the sum of all two digit numbers which when divided by 4, yields 1 as remainder.
How many terms of the A.P. −6, \[- \frac{11}{2}\], −5, ... are needed to give the sum −25?
If a, b, c is in A.P., prove that:
(a − c)2 = 4 (a − b) (b − c)
If a, b, c is in A.P., prove that:
a3 + c3 + 6abc = 8b3.
The income of a person is Rs 300,000 in the first year and he receives an increase of Rs 10000 to his income per year for the next 19 years. Find the total amount, he received in 20 years.
We know that the sum of the interior angles of a triangle is 180°. Show that the sums of the interior angles of polygons with 3, 4, 5, 6, ... sides form an arithmetic progression. Find the sum of the interior angles for a 21 sided polygon.
Write the common difference of an A.P. the sum of whose first n terms is
If the sum of n terms of an AP is 2n2 + 3n, then write its nth term.
If \[\frac{3 + 5 + 7 + . . . + \text { upto n terms }}{5 + 8 + 11 + . . . . \text { upto 10 terms }}\] 7, then find the value of n.
In n A.M.'s are introduced between 3 and 17 such that the ratio of the last mean to the first mean is 3 : 1, then the value of n is
If the sum of n terms of an A.P. is 2 n2 + 5 n, then its nth term is
Mark the correct alternative in the following question:
The 10th common term between the A.P.s 3, 7, 11, 15, ... and 1, 6, 11, 16, ... is
If second, third and sixth terms of an A.P. are consecutive terms of a G.P., write the common ratio of the G.P.
If 9 times the 9th term of an A.P. is equal to 13 times the 13th term, then the 22nd term of the A.P. is ______.
Any term of an A.P. (except first) is equal to half the sum of terms which are equidistant from it.
If b2, a2, c2 are in A.P., then `1/(a + b), 1/(b + c), 1/(c + a)` will be in ______
