Advertisements
Advertisements
प्रश्न
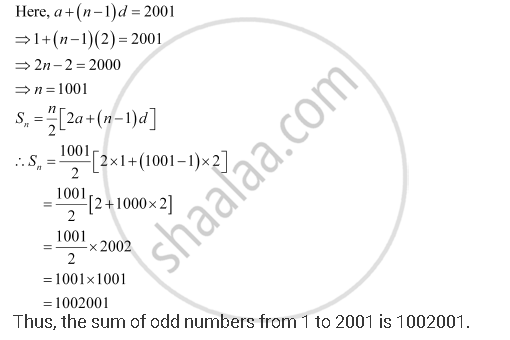
Find the sum of odd integers from 1 to 2001.
उत्तर
The odd integers from 1 to 2001 are 1, 3, 5, …1999, 2001.
This sequence forms an A.P.
Here, first term, a = 1
Common difference, d = 2
APPEARS IN
संबंधित प्रश्न
If the sum of a certain number of terms of the A.P. 25, 22, 19, … is 116. Find the last term
If the sum of n terms of an A.P. is (pn + qn2), where p and q are constants, find the common difference.
Between 1 and 31, m numbers have been inserted in such a way that the resulting sequence is an A.P. and the ratio of 7th and (m – 1)th numbers is 5:9. Find the value of m.
If the sum of three numbers in A.P., is 24 and their product is 440, find the numbers.
if `a(1/b + 1/c), b(1/c+1/a), c(1/a+1/b)` are in A.P., prove that a, b, c are in A.P.
A person writes a letter to four of his friends. He asks each one of them to copy the letter and mail to four different persons with instruction that they move the chain similarly. Assuming that the chain is not broken and that it costs 50 paise to mail one letter. Find the amount spent on the postage when 8th set of letter is mailed.
Find:
nth term of the A.P. 13, 8, 3, −2, ...
If the sequence < an > is an A.P., show that am +n +am − n = 2am.
Which term of the A.P. 84, 80, 76, ... is 0?
Is 68 a term of the A.P. 7, 10, 13, ...?
How many terms are there in the A.P. 7, 10, 13, ... 43 ?
The first term of an A.P. is 5, the common difference is 3 and the last term is 80; find the number of terms.
How many numbers are there between 1 and 1000 which when divided by 7 leave remainder 4?
Find the sum of the following arithmetic progression :
a + b, a − b, a − 3b, ... to 22 terms
Find the sum of first n odd natural numbers.
Find the sum of all integers between 50 and 500 which are divisible by 7.
Find the sum of all those integers between 100 and 800 each of which on division by 16 leaves the remainder 7.
If Sn = n2 p and Sm = m2 p, m ≠ n, in an A.P., prove that Sp = p3.
Find the sum of all two digit numbers which when divided by 4, yields 1 as remainder.
If a, b, c is in A.P., prove that:
a3 + c3 + 6abc = 8b3.
Insert five numbers between 8 and 26 such that the resulting sequence is an A.P.
A man starts repaying a loan as first instalment of Rs 100 = 00. If he increases the instalments by Rs 5 every month, what amount he will pay in the 30th instalment?
A carpenter was hired to build 192 window frames. The first day he made five frames and each day thereafter he made two more frames than he made the day before. How many days did it take him to finish the job?
A man saved ₹66000 in 20 years. In each succeeding year after the first year he saved ₹200 more than what he saved in the previous year. How much did he save in the first year?
If \[\frac{3 + 5 + 7 + . . . + \text { upto n terms }}{5 + 8 + 11 + . . . . \text { upto 10 terms }}\] 7, then find the value of n.
The first and last term of an A.P. are a and l respectively. If S is the sum of all the terms of the A.P. and the common difference is given by \[\frac{l^2 - a^2}{k - (l + a)}\] , then k =
Mark the correct alternative in the following question:
If in an A.P., the pth term is q and (p + q)th term is zero, then the qth term is
Mark the correct alternative in the following question:
Let Sn denote the sum of first n terms of an A.P. If S2n = 3Sn, then S3n : Sn is equal to
If for an arithmetic progression, 9 times nineth term is equal to 13 times thirteenth term, then value of twenty second term is ____________.
The pth term of an A.P. is a and qth term is b. Prove that the sum of its (p + q) terms is `(p + q)/2[a + b + (a - b)/(p - q)]`.
If the sum of m terms of an A.P. is equal to the sum of either the next n terms or the next p terms, then prove that `(m + n) (1/m - 1/p) = (m + p) (1/m - 1/n)`
If a, b, c, d are four distinct positive quantities in A.P., then show that bc > ad
The first term of an A.P.is a, and the sum of the first p terms is zero, show that the sum of its next q terms is `(-a(p + q)q)/(p - 1)`
A man saved Rs 66000 in 20 years. In each succeeding year after the first year he saved Rs 200 more than what he saved in the previous year. How much did he save in the first year?
If 9 times the 9th term of an A.P. is equal to 13 times the 13th term, then the 22nd term of the A.P. is ______.
Let 3, 6, 9, 12 ....... upto 78 terms and 5, 9, 13, 17 ...... upto 59 be two series. Then, the sum of the terms common to both the series is equal to ______.
If the ratio of the sum of n terms of two APs is 2n:(n + 1), then the ratio of their 8th terms is ______.
The sum of n terms of an AP is 3n2 + 5n. The number of term which equals 164 is ______.
If 100 times the 100th term of an A.P. with non zero common difference equals the 50 times its 50th term, then the 150th term of this A.P. is ______.
