Advertisements
Advertisements
प्रश्न
If tan θ = `1/sqrt(7) `show that ` (cosec ^2 θ - sec^2 θ)/(cosec^2 θ + sec^2 θ ) = 3/4`
उत्तर
Let us consider a right ΔABC, right angled at B and ∠𝐶 = 𝜃.
Now it is given that tan 𝜃 = `(AB)/(BC) = 1/(sqrt(7))`
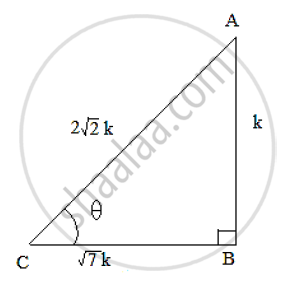
So, if AB = k, then BC = `sqrt(7) `𝑘, 𝑤ℎ𝑒𝑟 𝑘 𝑖𝑠 𝑎 𝑝𝑜𝑠𝑖𝑡𝑖𝑣𝑒 𝑛𝑢𝑚𝑏𝑒𝑟.
Using Pythagoras theorem, we have:
`AC^2 = AB^2 + BC^2`
`⟹ AC^2 = (k)^2 + (sqrt(7K)`
`⟹ AC^2 = K^2 + 7K^2`
`⟹ AC = 2sqrt(2K)`
Now, finding out the values of the other trigonometric ratios, we have:
sin θ = `(AB)/(AC) = K/(2sqrt(2k)) = 1/(2sqrt(2))`
cos θ = `( BC)/(AC) = (sqrt(7k))/(2 sqrt(2k)) = (sqrt(7))/(2sqrt(2))`
∴ 𝑐𝑜𝑠𝑒𝑐 𝜃 =`1/(sin θ) = 2 sqrt(2) and sec θ = 1/cos θ = (2sqrt(2))/(sqrt(7))`
Substituting the values of cosec θ and sec θ in the give expression, we get:
`(cosec^2 θ - sec^2 θ)/(cosec^2 θ + sec2 θ)`
=`(2 sqrt(2)^2-((2sqrt(2))/sqrt(7))^2)/(2sqrt({2)^2)+((2sqrt(2))/sqrt(7))^2)`
=`(8-(8/7))/(8+(8/7))`
= `((56-8)/7)/((56+8)/7)`
=`48/64 = 3/4 `= 𝑅𝐻𝑆
i.e., LHS = RHS
Hence proved.
APPEARS IN
संबंधित प्रश्न
In Fig below, Find tan P and cot R. Is tan P = cot R?

If `sin A = 9/41` compute cos 𝐴 𝑎𝑛𝑑 tan 𝐴
if `sec A = 5/4` verify that `(3 sin A - 4 sin^3 A)/(4 cos^3 A - 3 cos A) = (3 tan A - tan^3 A)/(1- 3 tan^2 A)`
In ∆PQR, right-angled at Q, PQ = 3 cm and PR = 6 cm. Determine ∠P and ∠R.
If sin θ = cos (θ – 45°), where θ – 45° are acute angles, find the degree measure of θ
If 2θ + 45° and 30° − θ are acute angles, find the degree measure of θ satisfying Sin (20 + 45°) = cos (30 - θ°)
Verify each of the following:
(ii)`cos 60^0 cos 30^0+ sin 60^0 sin30^0`
If 3x = cosecθ = and `3/x= cottheta` find the value of 3`(x^2-1/x^2)`.
Given: sec A = `( 29 )/(21), "evaluate : sin A" - 1/tan "A"`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cosB = `(4)/(5)`
