Advertisements
Advertisements
प्रश्न
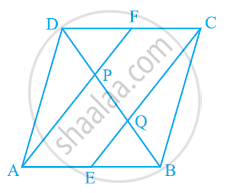
In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively (see the given figure). Show that the line segments AF and EC trisect the diagonal BD.
उत्तर
ABCD is a parallelogram.
∴ AB || CD
And hence, AE || FC
Again, AB = CD ...(Opposite sides of parallelogram ABCD)
`1/2 AB` = `1/2 CD`
AE = FC ...(E and F are mid-points of side AB and CD)
In quadrilateral AECF, one pair of opposite sides (AE and CF) is parallel and equal to each other. Therefore, AECF is a parallelogram.
⇒ AF || EC ...(Opposite sides of a parallelogram)
In ΔDQC, F is the mid-point of side DC and FP || CQ (as AF || EC). Therefore, by using the converse of mid-point theorem, it can be said that P is the mid-point of DQ.
⇒ DP = PQ ...(1)
Similarly, in ΔAPB, E is the mid-point of side AB and EQ || AP (as AF || EC). Therefore, by using the converse of mid-point theorem, it can be said that Q is the mid-point of PB.
⇒ PQ = QB ...(2)
From equations (1) and (2),
DP = PQ = BQ
Hence, the line segments AF and EC trisect the diagonal BD.
APPEARS IN
संबंधित प्रश्न
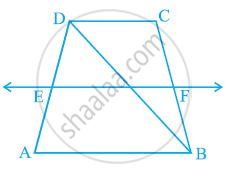
ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F (see the given figure). Show that F is the mid-point of BC.
In a triangle ∠ABC, ∠A = 50°, ∠B = 60° and ∠C = 70°. Find the measures of the angles of
the triangle formed by joining the mid-points of the sides of this triangle.
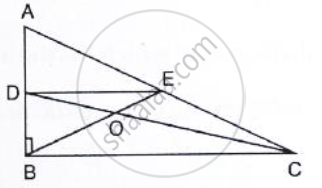
In Fig. below, triangle ABC is right-angled at B. Given that AB = 9 cm, AC = 15 cm and D,
E are the mid-points of the sides AB and AC respectively, calculate
(i) The length of BC (ii) The area of ΔADE.
ABC is a triangle and through A, B, C lines are drawn parallel to BC, CA and AB respectively
intersecting at P, Q and R. Prove that the perimeter of ΔPQR is double the perimeter of
ΔABC
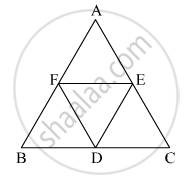
In the given figure, ΔABC is an equilateral traingle. Points F, D and E are midpoints of side AB, side BC, side AC respectively. Show that ΔFED is an equilateral traingle.
The diagonals of a quadrilateral intersect at right angles. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is rectangle.
L and M are the mid-point of sides AB and DC respectively of parallelogram ABCD. Prove that segments DL and BM trisect diagonal AC.
In triangle ABC, D and E are points on side AB such that AD = DE = EB. Through D and E, lines are drawn parallel to BC which meet side AC at points F and G respectively. Through F and G, lines are drawn parallel to AB which meets side BC at points M and N respectively. Prove that: BM = MN = NC.
In ΔABC, D, E, F are the midpoints of BC, CA and AB respectively. Find ∠FDB if ∠ACB = 115°.
E is the mid-point of the side AD of the trapezium ABCD with AB || DC. A line through E drawn parallel to AB intersect BC at F. Show that F is the mid-point of BC. [Hint: Join AC]
