Advertisements
Advertisements
प्रश्न
In a right-angled triangle ABC, ∠B = 90°, BD = 3, DC = 4, and AC = 13. A point D is inside the triangle such as ∠BDC = 90°.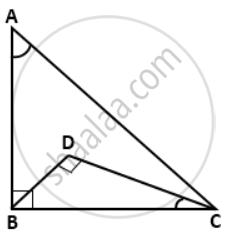
Find the values of 2 tan ∠BAC - sin ∠BCD
उत्तर
ΔBDC is a right-angled triangle.
∴ BC2
= BD2 +DC2
= 32 + 42
= 9 + 16
= 25
⇒ BC = 5cm
ΔABC is a right-angled triangle.
∴ AB2
= AC2 - BC2
= 132 - 52
= 169 - 25
= 144
⇒ AB = 12cm
2 tan ∠BAC - sin ∠BCD
= `2 xx "BC"/"AB" - "BD"/"BC"`
= `2 xx (5)/(12) - (3)/(5)`
= `(5)/(6) - (3)/(5)`
= `(25 - 18)/(30)`
= `(7)/(30)`.
APPEARS IN
संबंधित प्रश्न
In tan θ = 1, find the value of 5cot2θ + sin2θ - 1.
In the given figure, AD is perpendicular to BC. Find: 15 tan y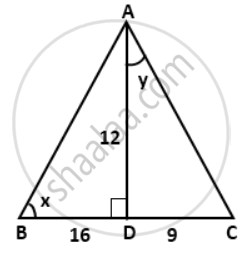
In a right-angled triangle ABC, ∠B = 90°, BD = 3, DC = 4, and AC = 13. A point D is inside the triangle such as ∠BDC = 90°.
Find the values of 3 - 2 cos ∠BAC + 3 cot ∠BCD
If 24cosθ = 7 sinθ, find sinθ + cosθ.
If 5cosθ = 3, find the value of `(4cosθ - sinθ)/(2cosθ + sinθ)`
If cotθ = `(1)/sqrt(3)`, show that `(1 - cos^2θ)/(2 - sin^2θ) = (3)/(5)`
If a cotθ = b, prove that `("a"sinθ - "b"cosθ)/("a"sinθ + "b"cosθ) = ("a"^2 - "b"^2)/("a"^2 + "b"^2)`
If secA = `(5)/(4)`, cerify that `(3sin"A" - 4sin^3"A")/(4cos^3"A" - 3cos"A") = (3tan"A" - tan^3"A")/(1 - 3tan^2"A")`.
If sinθ = `(3)/(4)`, prove that `sqrt(("cosec"^2θ - cot^2θ)/(sec^2θ - 1)) = sqrt(7)/(3)`.
If secA = `(17)/(8)`, verify that `(3 - 4sin^2 "A")/(4 cos^2 "A" - 3)= (3 - tan^2"A")/(1 - 3tan^2"A")`
