Advertisements
Advertisements
प्रश्न
In figure, if PQRS is a parallelogram and AB || PS, then prove that OC || SR.
उत्तर
According to the question,
PQRS is a parallelogram,
Therefore, PQ || SR and PS || QR.
Also given, AB || PS.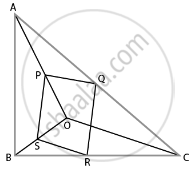
To prove:
OC || SR
From ∆OPS and OAB,
PS || AB
∠POS = ∠AOB ...[Common angle]
∠OSP = ∠OBA ...[Corresponding angles]
∴ ∆OPS ∼ ∆OAB ...[By AAA similarity criteria]
Then,
Using basic proportionality theorem,
We get,
`("PS")/("AB") = ("OS")/("OB")` ...(i)
From ∆CQR and ∆CAB,
QR || PS || AB
∠QCR = ∠ACB ...[Common angle]
∠CRQ = ∠CBA ...[Corresponding angles]
∴ ∆CQR ∼ ∆CAB
Then, by basic proportionality theorem
`("QR")/("AB") = ("CR")/("CB")`
⇒ `("PS")/("AB") = ("CR")/("CB")` ...(ii) [PS ≅ QR Since, PQRS is a parallelogram]
From equations (i) and (ii),
`("OS")/("OB") = ("CR")/("CB")`
or
`("OB")/("OS") = ("CB")/("CR")`
Subtracting 1 from L.H.S and R.H.S, we get,
`("OB")/("OS") - 1 = ("CB")/("CR") - 1`
⇒ `("OB" - "OS")/("OS") = ("CB" - "CR")/("CR")`
⇒ `("BS")/("OS") = ("BR")/("CR")`
SR || OC ...[By converse of basic proportionality theorem]
Hence proved.
APPEARS IN
संबंधित प्रश्न
Through the mid-point M of the side CD of a parallelogram ABCD, the line BM is drawn intersecting AC in L and AD produced in E. Prove that EL = 2 BL
In figure, ABCD is a trapezium with AB || DC. If ∆AED is similar to ∆BEC, prove that AD = BC.
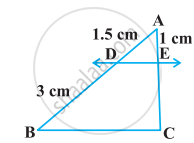
See the given figure. DE || BC. Find EC.
In the following figure, A, B and C are points on OP, OQ and OR respectively such that AB || PQ and AC || PR. Show that BC || QR.

In the given triangle P, Q and R are the mid-points of sides AB, BC and AC respectively. Prove that triangle PQR is similar to triangle ABC.

In each of the given pairs of triangles, find which pair of triangles are similar. State the similarity criterion and write the similarity relation in symbolic form:

ABCD is parallelogram and E is a point on BC. If the diagonal BD intersects AE at F, prove that AF × FB = EF × FD.

The areas of two similar triangles are 169cm2 and 121cm2 respectively. If the longest side of the larger triangle is 26cm, find the longest side of the smaller triangle.
State the SSS-similarity criterion for similarity of triangles
In the given figure, A – D – C and B – E – C seg DE || side AB If AD = 5, DC = 3, BC = 6.4 then Find BE.

Δ ABC - Δ XYZ. If area of Δ ABC is 9cm2 and area of Δ XYZ is 16cm2 and if BC= 2.1cm, find the length of YZ.
Δ ABC ∼ Δ PQR such that AB= 1.5 cm and PQ=2. 1 cm. Find the ratio of areas of Δ ABC and ΔPQR.
A model of a ship is made with a scale factor of 1 : 500. Find
The deck area of the model, if the deck area of the ship is 1500000 m2
On a map drawn to a scale of 1 : 25000, a rectangular plot of land, ABCD is measured as AB= 12 cm and BC = 16cm. calculate the diagonal distance of the plot in km and the plot area in km2 .
A model of an aeroplane is made to a scale of 1 : 400. Calculate : the length, in cm, of the model; if the length of the aeroplane is 40 m.
A model of an aeroplane is made to a scale of 1 : 400. Calculate : the length, in m, of the aeroplane, if length of its model is 16 cm.
On a map drawn to a scale of 1 : 2,50,000; a triangular plot of land has the following measurements : AB = 3 cm, BC = 4 cm and ∠ABC = 90°.
Calculate : the actual lengths of AB and BC in km.
A model of a ship is made to a scale 1 : 300.
- The length of the model of the ship is 2 m. Calculate the length of the ship.
- The area of the deck of the ship is 180,000 m2. Calculate the area of the deck of the model.
- The volume of the model is 6.5 m3. Calculate the volume of the ship.
In the following figure, point D divides AB in the ratio 3 : 5. Find : `(AD)/(AB)`

Choose the correct alternative:
If ΔABC ~ ΔPQR and 4A (ΔABC) = 25 A(ΔPQR), then AB : PQ = ?
In the given figure, PB is the bisector of ABC and ABC =ACB. Prove that:
a. BC x AP = PC x AB
b. AB:AC = BP: BC
Find the scale factor in each of the following and state the type of size transformation:
Model volume = 200cm3, Actual volume = 8cm3
The scale of a map is 1 : 50000. The area of a city is 40 sq km which is to be represented on the map. Find: The length of a scale in km represented by 1cm on the map.
If ∆ABC ~ ∆DEF such that area of ∆ABC is 9 cm2 and the area of ∆DEF is 16 cm2 and BC = 2.1 cm. Find the length of EF.
Construct a triangle similar to a given triangle LMN with its sides equal to `4/5` of the corresponding sides of the triangle LMN (scale factor `4/5 < 1`)
Construct a triangle similar to a given triangle PQR with its sides equal to `7/3` of the corresponding sides of the triangle PQR (scale factor `7/3 > 1`)
If ∆ABC – ∆PQR in which ∠A = 53° and ∠Q = 77°, then ∠R is
Areas of two similar triangles are 225 cm2 and 81 cm2. If side of smaller triangle is 12 cm, find corresponding side of major triangle.
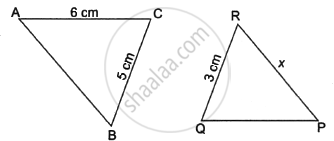
In the given figure, ΔABC ∼ ΔQPR, If AC = 6 cm, BC = 5 cm, QR = 3 cm and PR = x; them the value of x is ______.