Advertisements
Advertisements
प्रश्न
In right angled triangle ABC. ∠C = 90°, ∠B = 60°. AB = 15 units. Find remaining angles and sides.
उत्तर १
We are given the following triangle with related information
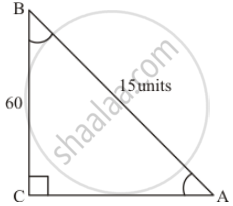
It is required to find ∠A, ∠C and length of sides AC and BC
ΔABC is right angled at C
Therefore,
`∠C = 90^@`
Now we know that sum of all the angles of any triangle is `180^@`
Therefore
`∠A + ∠B + ∠C = 180^@`
Now by substituting the values of known angles and in equation (1)
We get,
`∠A + 60^@ + 90^2 = 180^@`
Therefore
`∠A + 150^@ = 180^@`
`=> ∠A = 180^@ - 150^@`
`=> ∠A = 30^@`
Therefore,
`∠A = 30^@`
Now
We know that,
`cos B = cos 60^@`
`=> (BC)/(AB) = cos 60^@`
Now we have,
AB =15 units and `cos 60^@ = 1/2`
Therefore by substituting above values in equation (2)
We get,
`cos B = cos 60^@`
`=> (BC)/(AB) = cos 60^@`
`=> (BC)/15 = 1/2`
Now by cross multiplying we get,
`(BC)/15 = 1/2`
`=> 2 xx BC = 15 xx 1`
`=> BC = 15/2`
=> BC = 7.5
Therefore
BC = 7.5 units ....(3)
Now
We know that
`sin B = sin 60^@`
`=> (AC)/(AB) = sin 60^@` .........(4)
Now
We know that,
AB=15 units and
उत्तर २
We are given the following triangle with related information

It is required to find ∠A, ∠C and length of sides AC and BC
ΔABC is right angled at C
Therefore,
`∠C = 90^@`
Now we know that sum of all the angles of any triangle is `180^@`
Therefore
`∠A + ∠B + ∠C = 180^@`
Now by substituting the values of known angles and in equation (1)
We get,
`∠A + 60^@ + 90^2 = 180^@`
Therefore
`∠A + 150^@ = 180^@`
`=> ∠A = 180^@ - 150^@`
`=> ∠A = 30^@`
Therefore,
`∠A = 30^@`
Now
We know that,
`cos B = cos 60^@`
`=> (BC)/(AB) = cos 60^@`
Now we have,
AB =15 units and `cos 60^@ = 1/2`
Therefore by substituting above values in equation (2)
We get,
`cos B = cos 60^@`
`=> (BC)/(AB) = cos 60^@`
`=> (BC)/15 = 1/2`
Now by cross multiplying we get,
`(BC)/15 = 1/2`
`=> 2 xx BC = 15 xx 1`
`=> BC = 15/2`
=> BC = 7.5
Therefore
BC = 7.5 units ....(3)
Now
We know that
`sin B = sin 60^@`
`=> (AC)/(AB) = sin 60^@` .........(4)
Now
We know that,
`AB=15 units and sin 60^@ = sqrt3/2`
Therefore by substituting above values in equation (4)
We get,
`sin B = sin 60^@`
`=> (AC)/(AB) = sin 60^@`
`=> (AC)/15 = sqrt3/2`
Now by cross multiplying we get,
`=> 2 xx AC = sqrt3 xx 15`
`=> AC = (sqrt3 x 15)/2`
`=> AC = 15/2 sqrt3`
Therefore,
AC = 15/2 sqrt3` units`
Hence
`A = 30^@`
`BC = 7.5untis`
`AC = 15/2 sqrt3` units
APPEARS IN
संबंधित प्रश्न
If sin θ = cos (θ – 45°), where θ – 45° are acute angles, find the degree measure of θ
If sin θ = ` (a^2 - b^2)/(a^2+b^2)`find all the values of all T-ratios of θ .
If cos θ = `3/5` , show that `((sin theta - cot theta ))/(2tan theta)=3/160`
Using the formula, tan 2A =`(2 tan A )/(1- tan^2 A)` find the value of tan 600, it being given that tan 300 = `1/sqrt(3)`.
In a right-angled triangle, it is given that A is an acute angle and tan A = `(5) /(12)`.
find the value of :
(i) cos A
(ii) sin A
(iii) ` (cosA+sinA)/(cosA– sin A)`
Using the measurements given in the following figure:
(i) Find the value of sin θ and tan θ.
(ii) Write an expression for AD in terms of θ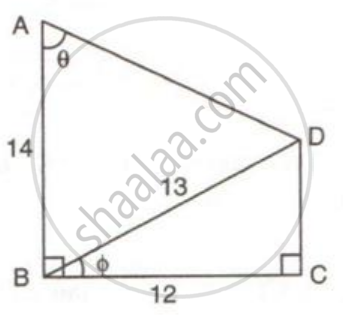
If sin A = cos A, find the value of 2 tan2A - 2 sec2 A + 5.
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: tan A
If 2 cos θ = `sqrt(3)`, then find all the trigonometric ratios of angle θ
If cos A = `3/5`, then find the value of `(sin"A" - cos"A")/(2tan"A")`
