Advertisements
Advertisements
प्रश्न
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(vii) f2 + 7f
उत्तर
Given:
\[f\left( x \right) = \sqrt{x + 1}\text{ and } g\left( x \right) = \sqrt{9 - x^2}\]
Clearly,
Thus, domain (f) = [1, ∞]
Again,
⇒ \[x \in \left[ - 3, 3 \right]\]
(vii) \[f^2 + 7f: \left[ - 1, \infty \right] \to \text{ R is given by } \left( f^2 + 7f \right)\left( x \right) = f^2 \left( x \right) + 7f\left( x \right)\] {Since domain(f) = [ - 1, ∞]}
APPEARS IN
संबंधित प्रश्न
Let f be the subset of Z × Z defined by f = {(ab, a + b): a, b ∈ Z}. Is f a function from Z to Z: justify your answer.
A function f : R → R is defined by f(x) = x2. Determine (a) range of f, (b) {x : f(x) = 4}, (c) [y: f(y) = −1].
If f(x) = x2 − 3x + 4, then find the values of x satisfying the equation f(x) = f(2x + 1).
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(vi) \[2f - \sqrt{5} g\]
Let A and B be two sets such that n(A) = p and n(B) = q, write the number of functions from A to B.
The range of f(x) = cos [x], for π/2 < x < π/2 is
Which of the following are functions?
If \[f\left( x \right) = \frac{\sin^4 x + \cos^2 x}{\sin^2 x + \cos^4 x}\] for x ∈ R, then f (2002) =
Check if the following relation is function:
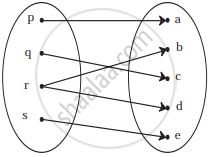
If ƒ(m) = m2 − 3m + 1, find f(x + 1)
If f(x) = `{(x^2 + 3"," x ≤ 2),(5x + 7"," x > 2):},` then find f(0)
Which sets of ordered pairs represent functions from A = {1, 2, 3, 4} to B = {−1, 0, 1, 2, 3}? Justify.
{(1, 0), (3, 3), (2, −1), (4, 1), (2, 2)}
Check if the relation given by the equation represents y as function of x:
3x − 6 = 21
If f(m) = m2 − 3m + 1, find `(("f"(2 + "h") - "f"(2))/"h"), "h" ≠ 0`
Find the domain and range of the following function.
f(x) = `root(3)(x + 1)`
Check the injectivity and surjectivity of the following function.
f : Z → Z given by f(x) = x2
Show that if f : A → B and g : B → C are onto, then g ° f is also onto
Express the following exponential equation in logarithmic form
`9^(3/2)` = 27
Express the following exponential equation in logarithmic form
e–x = 6
Express the following logarithmic equation in exponential form
ln e = 1
If f(x) = ax2 − bx + 6 and f(2) = 3 and f(4) = 30, find a and b
Solve for x.
log2 + log(x + 3) – log(3x – 5) = log3
Solve for x.
2 log10 x = `1 + log_10 (x + 11/10)`
If f(x) = 3x + 5, g(x) = 6x − 1, then find (fg) (3)
If f(x) = 3x + 5, g(x) = 6x − 1, then find `("f"/"g") (x)` and its domain
Select the correct answer from given alternatives.
If log (5x – 9) – log (x + 3) = log 2 then x = ...............
Answer the following:
Identify the following relation is the function? If it is a function determine its domain and range.
{(2, 1), (4, 2), (6, 3), (8, 4), (10, 5), (12, 6), (14, 7)}
Answer the following:
A function f : R → R defined by f(x) = `(3x)/5 + 2`, x ∈ R. Show that f is one-one and onto. Hence find f–1
Answer the following:
If f(x) = ax2 + bx + 2 and f(1) = 3, f(4) = 42, find a and b
Answer the following:
Simplify, log (log x4) – log (log x)
Answer the following:
Solve for x, logx (8x – 3) – logx 4 = 2
Answer the following:
Show that, logy x3 . logz y4 . logx z5 = 60
Answer the following:
Find the domain of the following function.
f(x) = 5–xPx–1
Find the domain for which the functions f(x) = 2x2 – 1 and g(x) = 1 – 3x are equal.
The value of the function f(x) = `(x^2 - 3x + 2)/(x^2 + x - 6)` lies in the interval
The domain of the function f(x) = `sin^-1((|x| + 5)/(x^2 + 1))` is (–∞, –a] ≈ [a, ∞). Then a is equal to ______.
The ratio `(2^(log_2 1/4 a) - 3^(log_27(a^2 + 1)^3) - 2a)/(7^(4log_49a) - a - 1)` simplifies to ______.
Let f(θ) = sin θ (sin θ + sin 3θ) then ______.
