Advertisements
Advertisements
प्रश्न
Read the following passage and answer the questions given below.
|
|
- Is the function differentiable in the interval (0, 12)? Justify your answer.
- If 6 is the critical point of the function, then find the value of the constant m.
- Find the intervals in which the function is strictly increasing/strictly decreasing.
OR
Find the points of local maximum/local minimum, if any, in the interval (0, 12) as well as the points of absolute maximum/absolute minimum in the interval [0, 12]. Also, find the corresponding local maximum/local minimum and the absolute ‘maximum/absolute minimum values of the function.
उत्तर
i. f(x) = –0.1x2 + mx + 98.6, being a polynomial function, is differentiable everywhere, hence, differentiable in (0, 12)
ii. f'(x) = –0.2x + m
Since, 6 is the critical point,
f'(6) = 0
⇒ m = 1.2
iii. f(x) = –0.1x2 + 1.2x + 98.6
f'(x) = –0.2x + 1.2 = –0.2(x – 6)
| In the Interval | f'(x) | Conclusion |
| (0, 6) | +ve | f is strictly increasing in [0, 6] |
| (6, 12) | -ve | f is strictly decreasing in [6, 12] |
OR
iii. f(x) = –0.1x2 + mx + 98.6,
f'(x) = –0.2x + 1.2, f'(6) = 0,
f"(x) = –0.2
f"(6) = –0.2 < 0
Hence, by second derivative test 6 is a point of local maximum. The local maximum value = f(6) = −0.1 × 62 + 1.2 × 6 + 98.6 = 102.2
We have f(0) = 98.6, f(6) = 102.2, f(12) = 98.6
6 is the point of absolute maximum and the absolute maximum value of the function = 102.2.
0 and 12 both are the points of absolute minimum and the absolute minimum value of the function = 98.6.
APPEARS IN
संबंधित प्रश्न
Examine the maxima and minima of the function f(x) = 2x3 - 21x2 + 36x - 20 . Also, find the maximum and minimum values of f(x).
Find the approximate value of cos (89°, 30'). [Given is: 1° = 0.0175°C]
Show that the height of the cylinder of maximum volume, that can be inscribed in a sphere of radius R is `(2R)/sqrt3.` Also, find the maximum volume.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
`g(x) = 1/(x^2 + 2)`
Find the local maxima and local minima, if any, of the following functions. Find also the local maximum and the local minimum values, as the case may be:
`f(x) = xsqrt(1-x), x > 0`
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
f (x) = sin x + cos x , x ∈ [0, π]
Show that of all the rectangles inscribed in a given fixed circle, the square has the maximum area.
Find the absolute maximum and minimum values of the function f given by f (x) = cos2 x + sin x, x ∈ [0, π].
Show that the surface area of a closed cuboid with square base and given volume is minimum, when it is a cube.
A given quantity of metal is to be cast into a half cylinder with a rectangular base and semicircular ends. Show that in order that the total surface area may be minimum the ratio of the length of the cylinder to the diameter of its semi-circular ends is \[\pi : (\pi + 2)\].
Find the point on the straight line 2x+3y = 6, which is closest to the origin.
Find the largest size of a rectangle that can be inscribed in a semicircle of radius 1 unit, so that two vertices lie on the diameter.
Find the volume of the largest cylinder that can be inscribed in a sphere of radius ‘r’ cm.
Solve the following : Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is `(2"R")/sqrt(3)`. Also, find the maximum volume.
The total cost of producing x units is ₹ (x2 + 60x + 50) and the price is ₹ (180 − x) per unit. For what units is the profit maximum?
The maximum and minimum values for the function f(x) = 4x3 - 6x2 on [-1, 2] are ______
If the sum of the lengths of the hypotenuse and a side of a right-angled triangle is given, show that the area of the triangle is maximum when the angle between them is `pi/3`
Find the points of local maxima, local minima and the points of inflection of the function f(x) = x5 – 5x4 + 5x3 – 1. Also find the corresponding local maximum and local minimum values.
The maximum value of `["x"("x" − 1) + 1]^(1/3)`, 0 ≤ x ≤ 1 is:
Find all the points of local maxima and local minima of the function f(x) = (x - 1)3 (x + 1)2
Find both the maximum and minimum values respectively of 3x4 - 8x3 + 12x2 - 48x + 1 on the interval [1, 4].
Let f(x) = 1 + 2x2 + 22x4 + …… + 210x20. Then f (x) has ____________.
Range of projectile will be maximum when angle of projectile is
The maximum value of the function f(x) = `logx/x` is ______.
The range of a ∈ R for which the function f(x) = `(4a - 3)(x + log_e5) + 2(a - 7)cot(x/2)sin^2(x/2), x ≠ 2nπ, n∈N` has critical points, is ______.
Let x and y be real numbers satisfying the equation x2 – 4x + y2 + 3 = 0. If the maximum and minimum values of x2 + y2 are a and b respectively. Then the numerical value of a – b is ______.
Let f(x) = (x – a)ng(x) , where g(n)(a) ≠ 0; n = 0, 1, 2, 3.... then ______.
A metal wire of 36 cm long is bent to form a rectangle. Find its dimensions when its area is maximum.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) `= x sqrt(1 - x), 0 < x < 1`
Sumit has bought a closed cylindrical dustbin. The radius of the dustbin is ‘r' cm and height is 'h’ cm. It has a volume of 20π cm3.
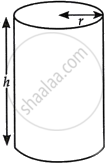
- Express ‘h’ in terms of ‘r’, using the given volume.
- Prove that the total surface area of the dustbin is `2πr^2 + (40π)/r`
- Sumit wants to paint the dustbin. The cost of painting the base and top of the dustbin is ₹ 2 per cm2 and the cost of painting the curved side is ₹ 25 per cm2. Find the total cost in terms of ‘r’, for painting the outer surface of the dustbin including the base and top.
- Calculate the minimum cost for painting the dustbin.

