Advertisements
Advertisements
प्रश्न
Show that the angles of an equilateral triangle are 60° each.
उत्तर
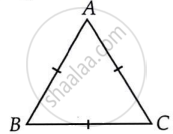
Let ABC be an equilateral triangle.
∴ AB = BC = AC …(A)
AB = BC ...[Taking first and second terms]
⇒ ∠C = ∠A …(i) ...[Angles opposite to equal sides]
Therefore,
AB = AC ...[Taking first and third terms of (A)]
⇒ ∠C = ∠B …(ii) ...[Angles opposite to equal sides]
From (i) and (ii) we get
∠A = ∠B = ∠C …(iii)
Now in △ABC …(iv)
∠A + ∠B + ∠C = 180° ...[Angle Sum Property]
⇒ ∠A + ∠A + ∠A = 180°
⇒ 3∠A = 180
⇒ ∠A = 60°
From (iii), ∠A = ∠B = ∠C
⇒ ∠A = ∠B = ∠C = 60°
Hence, each angle of an equilateral triangle is 60°.
APPEARS IN
संबंधित प्रश्न
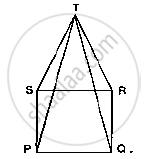
In Fig. 10.23, PQRS is a square and SRT is an equilateral triangle. Prove that
(i) PT = QT (ii) ∠TQR = 15°
BD and CE are bisectors of ∠B and ∠C of an isosceles ΔABC with AB = AC. Prove that BD = CE.
If the bisector of the exterior vertical angle of a triangle be parallel to the base. Show that the triangle is isosceles.
In a ΔABC, it is given that AB = AC and the bisectors of ∠B and ∠C intersect at O. If M is a point on BO produced, prove that ∠MOC = ∠ABC.
Which of the following statements are true (T) and which are false (F):
The bisectors of two equal angles of a triangle are equal
Which of the following statements are true (T) and which are false (F):
If the bisector of the vertical angle of a triangle bisects the base, then the triangle may be isosceles.
In a triangle ABC, if AB = AC and AB is produced to D such that BD = BC, find ∠ACD: ∠ADC.
If the measures of angles of a triangle are in the ratio of 3 : 4 : 5, what is the measure of the smallest angle of the triangle?
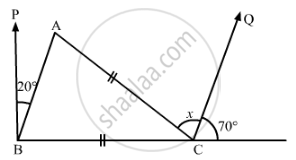
In the given figure, if BP || CQ and AC = BC, then the measure of x is
Two sides of a triangle are of lengths 5 cm and 1.5 cm. The length of the third side of the triangle cannot be ______.
