Advertisements
Advertisements
प्रश्न
The range of \[f\left( x \right) = \frac{1}{1 - 2\cos x}\] is
विकल्प
(a) [1/3, 1]
(b) [−1, 1/3]
(c) (−∞, −1) ∪ [1/3, ∞)
(d) [−1/3, 1]
उत्तर
We know that −1 ≤ cosx ≤ 1 for all x ∈ R.
Now,
\[- 1 \leq \cos x \leq 1\]
\[ \Rightarrow - 1 \leq - \cos x \leq 1\]
\[ \Rightarrow - 2 \leq - 2\cos x \leq 2\]
\[ \Rightarrow - 1 \leq 1 - 2\cos x \leq 3 \left( \text{ Adding 1 to each term } \right)\]
But,
\[ \Rightarrow \frac{1}{1 - 2\cos x} \in ( - \infty , - 1] \cup [\frac{1}{3}, \infty )\]
Disclaimer: The range of the function does not matches with either of the given options. The range matches with option (c) if it is given as "(−∞, −1] ∪ [1/3, ∞)".
APPEARS IN
संबंधित प्रश्न
Let A = {−2, −1, 0, 1, 2} and f : A → Z be a function defined by f(x) = x2 − 2x − 3. Find:
(b) pre-images of 6, −3 and 5.
A function f : R → R is defined by f(x) = x2. Determine (a) range of f, (b) {x : f(x) = 4}, (c) [y: f(y) = −1].
Let f : R+ → R, where R+ is the set of all positive real numbers, such that f(x) = loge x. Determine
(c) whether f(xy) = f(x) : f(y) holds
Let f : R → R and g : C → C be two functions defined as f(x) = x2 and g(x) = x2. Are they equal functions?
The function f is defined by \[f\left( x \right) = \begin{cases}x^2 , & 0 \leq x \leq 3 \\ 3x, & 3 \leq x \leq 10\end{cases}\]
The relation g is defined by \[g\left( x \right) = \begin{cases}x^2 , & 0 \leq x \leq 2 \\ 3x, & 2 \leq x \leq 10\end{cases}\]
Show that f is a function and g is not a function.
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(iv) \[\frac{g}{f}\] Also, find (f + g) (−1), (fg) (0),
Let f(x) = x2 and g(x) = 2x+ 1 be two real functions. Find (f + g) (x), (f − g) (x), (fg) (x) and \[\left( \frac{f}{g} \right) \left( x \right)\] .
Write the range of the real function f(x) = |x|.
If f(x) = cos (log x), then the value of f(x2) f(y2) −
If f(x) = cos (log x), then value of \[f\left( x \right) f\left( 4 \right) - \frac{1}{2} \left\{ f\left( \frac{x}{4} \right) + f\left( 4x \right) \right\}\] is
The domain of the function \[f\left( x \right) = \sqrt{\frac{\left( x + 1 \right) \left( x - 3 \right)}{x - 2}}\] is
The range of the function \[f\left( x \right) = \frac{x}{\left| x \right|}\] is
If \[\left[ x \right]^2 - 5\left[ x \right] + 6 = 0\], where [.] denotes the greatest integer function, then
A function f is defined as follows: f(x) = 5 − x for 0 ≤ x ≤ 4. Find the value of x such that f(x) = 3
If f(x) =` (2x−1)/ (5x−2) , x ≠ 2/5` Verify whether (fof) (x) = x
Check if the following relation is a function.
Find the domain and range of the follwoing function.
h(x) = `sqrt(x + 5)/(5 + x)`
Find the domain and range of the following function.
f(x) = `sqrt((x - 3)/(7 - x))`
Find the domain and range of the following function.
f(x) = `sqrt(16 - x^2)`
Show that if f : A → B and g : B → C are one-one, then g ° f is also one-one
Express the following exponential equation in logarithmic form
3–4 = `1/81`
Express the following logarithmic equation in exponential form
log10 (0.001) = −3
Solve for x.
x + log10 (1 + 2x) = x log10 5 + log10 6
If `log((x + y)/3) = 1/2 log x + 1/2 logy`, show that `x/y + y/x` = 7
Select the correct answer from given alternatives.
Find x, if 2log2 x = 4
Select the correct answer from given alternatives.
If f(x) =`1/(1 - x)`, then f{f[f(x)]} is
Answer the following:
A function f : R → R defined by f(x) = `(3x)/5 + 2`, x ∈ R. Show that f is one-one and onto. Hence find f–1
Answer the following:
Let f : R → R be given by f(x) = x3 + 1 for all x ∈ R. Draw its graph
Answer the following:
Simplify, log (log x4) – log (log x)
Answer the following:
Without using log tables, prove that `2/5 < log_10 3 < 1/2`
Answer the following:
Find the domain of the following function.
f(x) = `sqrt(x - x^2) + sqrt(5 - x)`
Answer the following:
Find the range of the following function.
f(x) = |x – 5|
Given the function f: x → x2 – 5x + 6, evaluate f(2)
Let f(x) = 2x + 5. If x ≠ 0 then find `(f(x + 2) -"f"(2))/x`
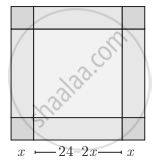
An open box is to be made from a square piece of material, 24 cm on a side, by cutting equal square from the corner and turning up the side as shown. Express the volume V of the box as a function of x
A function f is defined by f(x) = 3 – 2x. Find x such that f(x2) = (f(x))2
The domain of the function f(x) = log3+x (x2 - 1) is ______.
Let f : R → R be defined by
f(x) = `{(3x; x > 2),(2x^2; 1 ≤ x ≤ 2), (4x; x < 1):}`
Then f(-2) + f(1) + f(3) is ______
Let f(x) = `sqrt(x)` and g(x) = x be two functions defined in the domain R+ ∪ {0}. Find (fg)(x)
The value of the function f(x) = `(x^2 - 3x + 2)/(x^2 + x - 6)` lies in the interval
