Advertisements
Advertisements
प्रश्न
Answer the following:
Without using log tables, prove that `2/5 < log_10 3 < 1/2`
उत्तर
We have to show that, `2/5 < log_10 3 < 1/2`
i.e., to show that,
`2/5 < log_10 3` and `log_10 3 < 1/2`
i.e., to show that,
2 < 5log103 and 2 log103 < 1
i.e., to show that,
2 log1010 < 5 log103 and 2 log103 < log1010 ...[∵ log1010 = 1]
i.e., to show that,
log10102 < log1035 and log1032 < log1010
i.e., to show that,
102 < 35 and 32 < 10
i.e., to show that,
100 < 243 and 9 < 10
which is true
∴ `2/5 < log_10 3 < 1/2`.
APPEARS IN
संबंधित प्रश्न
Let A = {9, 10, 11, 12, 13} and let f: A → N be defined by f(n) = the highest prime factor of n. Find the range of f.
et A = (12, 13, 14, 15, 16, 17) and f : A → Z be a function given by
f(x) = highest prime factor of x.
Find range of f.
The function f is defined by \[f\left( x \right) = \begin{cases}x^2 , & 0 \leq x \leq 3 \\ 3x, & 3 \leq x \leq 10\end{cases}\]
The relation g is defined by \[g\left( x \right) = \begin{cases}x^2 , & 0 \leq x \leq 2 \\ 3x, & 2 \leq x \leq 10\end{cases}\]
Show that f is a function and g is not a function.
If \[f\left( x \right) = \begin{cases}x^2 , & \text{ when } x < 0 \\ x, & \text{ when } 0 \leq x < 1 \\ \frac{1}{x}, & \text{ when } x \geq 1\end{cases}\]
find: (a) f(1/2), (b) f(−2), (c) f(1), (d)
If \[f\left( x \right) = \frac{2x}{1 + x^2}\] , show that f(tan θ) = sin 2θ.
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(vii) f2 + 7f
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(i) f + g
If f(x) = loge (1 − x) and g(x) = [x], then determine function:
(iii) \[\frac{f}{g}\]
If f(x) = 4x − x2, x ∈ R, then write the value of f(a + 1) −f(a − 1).
The range of f(x) = cos [x], for π/2 < x < π/2 is
Which of the following are functions?
If f(x) = cos (log x), then value of \[f\left( x \right) f\left( 4 \right) - \frac{1}{2} \left\{ f\left( \frac{x}{4} \right) + f\left( 4x \right) \right\}\] is
If \[f\left( x \right) = \frac{2^x + 2^{- x}}{2}\] , then f(x + y) f(x − y) is equal to
If f : [−2, 2] → R is defined by \[f\left( x \right) = \begin{cases}- 1, & \text{ for } - 2 \leq x \leq 0 \\ x - 1, & \text{ for } 0 \leq x \leq 2\end{cases}\] , then
{x ∈ [−2, 2] : x ≤ 0 and f (|x|) = x} =
The domain of definition of the function \[f\left( x \right) = \sqrt{\frac{x - 2}{x + 2}} + \sqrt{\frac{1 - x}{1 + x}}\] is
The range of the function \[f\left( x \right) = \frac{x + 2}{\left| x + 2 \right|}\],x ≠ −2 is
If f(x) = `{(x^2 + 3"," x ≤ 2),(5x + 7"," x > 2):},` then find f(2)
Check if the following relation is a function.
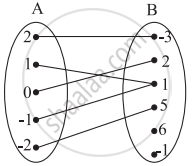
If f(m) = m2 − 3m + 1, find f(−3)
If f(m) = m2 − 3m + 1, find `f(1/2)`
Find x, if g(x) = 0 where g(x) = 6x2 + x − 2
Find x, if f(x) = g(x) where f(x) = x4 + 2x2, g(x) = 11x2
If f(x) = `("a" - x)/("b" - x)`, f(2) is undefined, and f(3) = 5, find a and b
Find the domain and range of the follwoing function.
h(x) = `sqrt(x + 5)/(5 + x)`
Check the injectivity and surjectivity of the following function.
f : R → R given by f(x) = x2
Express the following exponential equation in logarithmic form
10−2 = 0.01
Express the following exponential equation in logarithmic form
`"e"^(1/2)` = 1.6487
Express the following logarithmic equation in exponential form
`log_5 1/25` = – 2
Write the following expression as a single logarithm.
5 log x + 7 log y − log z
Prove that `"b"^(log_"b""a"` = a
Select the correct answer from given alternatives.
If log (5x – 9) – log (x + 3) = log 2 then x = ...............
Select the correct answer from given alternatives.
If f(x) =`1/(1 - x)`, then f{f[f(x)]} is
Answer the following:
Find the range of the following function.
f(x) = |x – 5|
Given the function f: x → x2 – 5x + 6, evaluate f(x – 1)
Redefine the function which is given by f(x) = `|x - 1| + |1 + x|, -2 ≤ x ≤ 2`
Find the domain of the following functions given by f(x) = `1/sqrt(x + |x|)`
Domain of `sqrt(a^2 - x^2) (a > 0)` is ______.
The domain of the function f defined by f(x) = `sqrt(4 - x) + 1/sqrt(x^2 - 1)` is equal to ______.
