Advertisements
Advertisements
प्रश्न
Using integration, find the area of the region bounded by line y = `sqrt(3)x`, the curve y = `sqrt(4 - x^2)` and Y-axis in first quadrant.
उत्तर
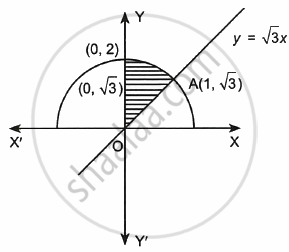
Given, y = `sqrt(4 - x^2)`
`\implies` x2 + y2 = 4
For finding point of intersection put y = `sqrt(3)x` in y = `sqrt(4 - x^2)`, we get
`sqrt(3)x = sqrt(4 - x^2)`
`\implies` 3x2 = 4 – x2
`\implies` 4x2 = 4
`\implies` x2 = 1
`\implies` x = ± 1
∴ y = `sqrt(3)`
∴ Coordinates of A is `(1, sqrt(3))`
∴ Required Area = `int_0^sqrt(3) y/sqrt(3) dy + int_sqrt(3)^2 sqrt(4 - y^2) dy`
= `1/sqrt(3) [y^2/2]_0^sqrt(3) + [y/2 sqrt(4 - y^2) + 4/2 sin^-1 (y/2)]_sqrt(3)^2`
= `1/sqrt(3) [3/2 - 0] + [2 sin^-1 (1) - sqrt(3)/2 - 2 sin^-1 (sqrt(3)/2)]`
= `sqrt(3)/2 + 2 xx π/2 - sqrt(3)/2 - 2 xx π/3`
= `π - (2π)/3`
= `π/3` sq. units.
APPEARS IN
संबंधित प्रश्न
Find the area of the region lying in the first quandrant bounded by the curve y2= 4x, X axis and the lines x = 1, x = 4
Find the area of ellipse `x^2/1 + y^2/4 = 1`
Using integration, find the area of the region bounded between the line x = 2 and the parabola y2 = 8x.
Draw a rough sketch to indicate the region bounded between the curve y2 = 4x and the line x = 3. Also, find the area of this region.
Find the area under the curve y = \[\sqrt{6x + 4}\] above x-axis from x = 0 to x = 2. Draw a sketch of curve also.
Draw a rough sketch of the graph of the function y = 2 \[\sqrt{1 - x^2}\] , x ∈ [0, 1] and evaluate the area enclosed between the curve and the x-axis.
Using integration, find the area of the region bounded by the line 2y = 5x + 7, x-axis and the lines x = 2 and x = 8.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Compare the areas under the curves y = cos2 x and y = sin2 x between x = 0 and x = π.
Find the area of the region bounded by x2 = 4ay and its latusrectum.
Find the area of the region bounded by the curve \[a y^2 = x^3\], the y-axis and the lines y = a and y = 2a.
Find the area of the region included between the parabola y2 = x and the line x + y = 2.
Find the area common to the circle x2 + y2 = 16 a2 and the parabola y2 = 6 ax.
OR
Find the area of the region {(x, y) : y2 ≤ 6ax} and {(x, y) : x2 + y2 ≤ 16a2}.
Find the area, lying above x-axis and included between the circle x2 + y2 = 8x and the parabola y2 = 4x.
Find the area of the region bounded by the curves y = x − 1 and (y − 1)2 = 4 (x + 1).
Find the area of the region enclosed by the parabola x2 = y and the line y = x + 2.
If the area above the x-axis, bounded by the curves y = 2kx and x = 0, and x = 2 is \[\frac{3}{\log_e 2}\], then the value of k is __________ .
The area bounded by the curve y2 = 8x and x2 = 8y is ___________ .
The area of the circle x2 + y2 = 16 enterior to the parabola y2 = 6x is
Find the area of the region bounded by the curves y2 = 9x, y = 3x
Find the area of the region included between y2 = 9x and y = x
Using integration, find the area of the region `{(x, y): 0 ≤ y ≤ sqrt(3)x, x^2 + y^2 ≤ 4}`
What is the area of the region bounded by the curve `y^2 = 4x` and the line `x` = 3.
Find the area of the region bounded by the curve `y = x^2 + 2, y = x, x = 0` and `x = 3`
Area (in sq.units) of the region outside `|x|/2 + |y|/3` = 1 and inside the ellipse `x^2/4 + y^2/9` = 1 is ______.
Let f(x) be a non-negative continuous function such that the area bounded by the curve y = f(x), x-axis and the ordinates x = `π/4` and x = `β > π/4` is `(βsinβ + π/4 cos β + sqrt(2)β)`. Then `f(π/2)` is ______.
Let g(x) = cosx2, f(x) = `sqrt(x)`, and α, β (α < β) be the roots of the quadratic equation 18x2 – 9πx + π2 = 0. Then the area (in sq. units) bounded by the curve y = (gof)(x) and the lines x = α, x = β and y = 0, is ______.
Using integration, find the area of the region bounded by the curve y2 = 4x and x2 = 4y.
