Advertisements
Advertisements
प्रश्न
Find the area of the region bounded by the curve \[a y^2 = x^3\], the y-axis and the lines y = a and y = 2a.
उत्तर
The equation of the given curve is \[a y^2 = x^3\]
The given curve passes through the origin. This curve is symmetrical about the x-axis.
The graph of the given curve is shown below.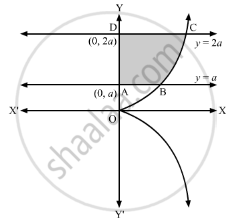
The lines y = a and y = 2a are parallel to the x-axis and intersects the y-axis at (0, a) and (0, 2a), respectively.
∴ Required area = Area of the shaded region
\[= \int_a^{2a} x_{\text{ curve }} dy\]
\[ = \int_a^{2a} \left( a y^2 \right)^\frac{1}{3} dy\]
\[ = a^\frac{1}{3} \int_a^{2a} y^\frac{2}{3} dy\]
\[ = \left.a^\frac{1}{3} \times \frac{y^\frac{5}{3}}{\frac{5}{3}}\right|_a^{2a} \]
\[ = \frac{3}{5} a^\frac{1}{3} \left[ \left( 2a \right)^\frac{5}{3} - a^\frac{5}{3} \right]\]
\[ = \frac{3}{5}\left( 2^\frac{5}{3} a^2 - a^2 \right)\]
\[ = \frac{3}{5}\left( 2^\frac{5}{3} - 1 \right) a^2\text{ square units }\]
APPEARS IN
संबंधित प्रश्न
Find the area of the sector of a circle bounded by the circle x2 + y2 = 16 and the line y = x in the ftrst quadrant.
Using integration, find the area of the region bounded by the lines y = 2 + x, y = 2 – x and x = 2.
Using the method of integration find the area of the region bounded by lines: 2x + y = 4, 3x – 2y = 6 and x – 3y + 5 = 0
Make a rough sketch of the graph of the function y = 4 − x2, 0 ≤ x ≤ 2 and determine the area enclosed by the curve, the x-axis and the lines x = 0 and x = 2.
Using integration, find the area of the region bounded by the line 2y = 5x + 7, x-axis and the lines x = 2 and x = 8.
Using definite integrals, find the area of the circle x2 + y2 = a2.
Draw a rough sketch of the curve \[y = \frac{x}{\pi} + 2 \sin^2 x\] and find the area between the x-axis, the curve and the ordinates x = 0 and x = π.
Find the area of the region bounded by x2 = 4ay and its latusrectum.
Using integration, find the area of the region bounded by the triangle whose vertices are (2, 1), (3, 4) and (5, 2).
Using integration, find the area of the region bounded by the triangle whose vertices are (−1, 2), (1, 5) and (3, 4).
Find the area of the region bounded by \[y = \sqrt{x}\] and y = x.
Find the area enclosed by the curve \[y = - x^2\] and the straight line x + y + 2 = 0.
Sketch the region bounded by the curves y = x2 + 2, y = x, x = 0 and x = 1. Also, find the area of this region.
Using integration, find the area of the triangle ABC coordinates of whose vertices are A (4, 1), B (6, 6) and C (8, 4).
Find the area of the region enclosed between the two curves x2 + y2 = 9 and (x − 3)2 + y2 = 9.
Find the area of the region bounded by the parabola y2 = 2x and the straight line x − y = 4.
The area bounded by the parabola x = 4 − y2 and y-axis, in square units, is ____________ .
The area bounded by the parabola y2 = 4ax, latusrectum and x-axis is ___________ .
The area of the region bounded by the parabola y = x2 + 1 and the straight line x + y = 3 is given by
The area bounded by the curve y2 = 8x and x2 = 8y is ___________ .
Find the equation of the standard ellipse, taking its axes as the coordinate axes, whose minor axis is equal to the distance between the foci and whose length of the latus rectum is 10. Also, find its eccentricity.
Find the area of the region bounded by the parabola y2 = 2x and the straight line x – y = 4.
Find the area of the region bounded by y = `sqrt(x)` and y = x.
Compute the area bounded by the lines x + 2y = 2, y – x = 1 and 2x + y = 7.
Find the area bounded by the lines y = 4x + 5, y = 5 – x and 4y = x + 5.
Find the area bounded by the curve y = 2cosx and the x-axis from x = 0 to x = 2π
Draw a rough sketch of the given curve y = 1 + |x +1|, x = –3, x = 3, y = 0 and find the area of the region bounded by them, using integration.
The area of the region bounded by the ellipse `x^2/25 + y^2/16` = 1 is ______.
Using integration, find the area of the region bounded between the line x = 4 and the parabola y2 = 16x.
Area lying in the first quadrant and bounded by the circle `x^2 + y^2 = 4` and the lines `x + 0` and `x = 2`.
Find the area of the region bounded by the ellipse `x^2/4 + y^2/9` = 1.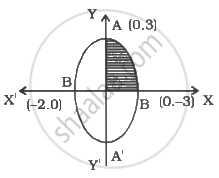
The area enclosed by y2 = 8x and y = `sqrt(2x)` that lies outside the triangle formed by y = `sqrt(2x)`, x = 1, y = `2sqrt(2)`, is equal to ______.
Let the curve y = y(x) be the solution of the differential equation, `("dy")/("d"x) = 2(x + 1)`. If the numerical value of area bounded by the curve y = y(x) and x-axis is `(4sqrt(8))/3`, then the value of y(1) is equal to ______.
Let T be the tangent to the ellipse E: x2 + 4y2 = 5 at the point P(1, 1). If the area of the region bounded by the tangent T, ellipse E, lines x = 1 and x = `sqrt(5)` is `sqrt(5)`α + β + γ `cos^-1(1/sqrt(5))`, then |α + β + γ| is equal to ______.
The area of the region S = {(x, y): 3x2 ≤ 4y ≤ 6x + 24} is ______.
Using integration, find the area of the region bounded by line y = `sqrt(3)x`, the curve y = `sqrt(4 - x^2)` and Y-axis in first quadrant.
Using integration, find the area bounded by the curve y2 = 4ax and the line x = a.
